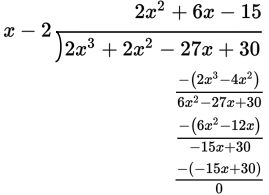Welcome to AMBiPi (Amans Maths Blogs). SAT (Scholastic Assessment Test) is a standard test, used for taking admission to undergraduate programs of universities or colleges in the United States. In this article, you will get SAT 2022 Math Study Practice Test 24 Grid Ins Questions with Answer Keys | SAT Online Course AMBiPi.
SAT 2022 Math Study Practice Test 24 Grid Ins Questions with Answer Keys
SAT Math Practice Online Test Question No 1:
11x – 24y = 8
kx – 36y = 5
In the system of equations above, k is a constant. If the system has no solution, what is the value of k?
Show/Hide Answer Key
Correct Answer: 16.5 or 33/2
Difficulty: Hard
Category: Heart of Algebra / Systems of Linear Equations
Strategic Advice: A system of linear equations that has no solution indicates two parallel lines (because parallel lines never intersect).
Getting to the Answer: Parallel lines have equal slopes, so rewrite each equation in slope-intercept form (y = mx + b) and set their slopes equal to each other:
11x – 24y = 8 → -24y = -11x + 8
→ y = (11/24)x – 8/24
kx – 36y = 5 → -36y = -kx + 5
→ y = (k/36)x – 5/36
The slope of the first line is 11/24 and the slope of the second line is k/36.
Set the slopes equal and cross-multiply to solve for k:
11/24 = k/36
24k = 11(36)
24k = 396
k = 16.5
Grid in 16.5 (or 33/2).
SAT Math Practice Online Test Question No 2:
Margo can peel and slice at least 10 dozen apples per hour and at most 15 dozen apples per hour. Based on this information, what is the possible amount of time, in hours, that it could take Margo to peel and slice 60 dozen apples?
Show/Hide Answer Key
Correct Answer: 4≤r≤6
Any value between 4 and 6, inclusive
Difficulty: Medium
Category: Heart of Algebra / Inequalities
Strategic Advice: When you see phrases like “at least” and “at most,” consider writing an inequality in words first and then translating from English into math. “At least” translates as ≥ because it means that much or more, and “at most” translates as ≤ because it means that much or less.
Getting to the Answer: Because Margo can peel and slice at least 10 dozen apples per hour and at most 15 dozen apples per hour, her rate is somewhere between 10 and 15 dozen apples per hour, or 10 ≤ r ≤ 15. This means her rate for 60 dozen apples is somewhere between 60 ÷ 10 = 6 hours and 60 ÷ 15 = 4 hours. Grid in any number between 4 and 6, inclusive.
SAT Math Practice Online Test Question No 3:
Eli left his home in New York and traveled to Brazil on business. Before he left, he used his credit card to purchase these pewter vases:

For daily purchases totaling less than 200 U.S. dollars, Eli’s credit card company charges a 2% fee. If the total charge on his credit card for the vases was $126.48, what was the foreign exchange rate in Brazilian reais (R$) per U.S. dollar on the day that Eli bought the vases? If necessary, round your answer to the nearest hundredth.
Show/Hide Answer Key
Correct Answer: 2.25
Difficulty: Hard
Category: Problem Solving and Data Analysis / Rates, Ratios, Proportions, and Percentages
Strategic Advice: Some questions, especially ones that are based on real-world scenarios, require a step-by-step approach. Make a plan and move through the plan one step at a time.
Getting to the Answer: Step 1: Find the total cost of the vases in Brazilian reais: 128 + 66 + 85 = 279.
Step 2: Find the total cost of the vases in U.S. dollars. The charge amount of $126.48 represents the conversion of 279 Brazilian reais plus the 2% fee that Eli’s credit card company charged him. To find the original cost, c, of the vases in U.S. dollars (before the 2% fee), write and solve the equation, 1.02c = 126.48. Dividing both sides of the equation by 1.02 results in a cost of c = $124.
Step 3: Find the exchange rate. To find the rate, r, in Brazilian reais per U.S. dollar, let the units guide you:
124 dollars x r reais/1 dollar = 279 reais
124r = 279
r = 279/124 = 2.25
The exchange rate that day was 2.25 Brazilian reais per U.S. dollar.
SAT Math Practice Online Test Question No 4:
A medical practice surveyed a random sample of 80 patients to determine whether their facility should open earlier in the morning or close later in the evening. Of the80 patients surveyed, 37.5% preferred that the facility open earlier. Based on this information, how many of the practice’s 600 patients would be expected to prefer that the facility open earlier in the morning?
Show/Hide Answer Key
Correct Answer: 225
Difficulty: Easy
Category: Problem Solving and Data Analysis / Statistics and Probability
Strategic Advice: When a random sample is used to survey part of a population, the results are fairly representative of the entire population.
Getting to the Answer: Because the medical practice surveyed a random sample, the percent of all the patients expected to prefer that the facility open earlier can be estimated by the percent of patients who preferred that option in the sample, 37.5%. Therefore, of the practice’s 600 patients, approximately 600 × 0.375 = 225 patients would be expected to prefer that the facility open earlier.
SAT Math Practice Online Test Question No 5:
If x = 5 and √2m + √11 – x = 0, what is the value of m?
Show/Hide Answer Key
Correct Answer: 7
Difficulty: Medium
Category: Passport to Advanced Math / Exponents
Strategic Advice: When you’re given the value of a variable in an equation, start by plugging in the given value and see where that takes you.
Getting to the Answer: Substitute 5 for x, isolate the radical term by adding 5 to both sides of the equation, square both sides to get rid of the square root, and continue using inverse operations until you have m by itself: √2m + √11 – 5 = 0
√2m + √11 = 5
(√2m + √11)2 = 52
2m + 11 = 25
2m = 14
m = 7
One final step-when you solve an equation that involves a square root, you must check that an extraneous (invalid) root was not produced. To check, plug 7 in for m (and the given 5 for x) into the original equation to make sure the result is a true statement:
√2(7) + √11 – 5 = √14 + √11 – 5
√25 – 5 = 5 – 5 = 0
SAT Math Practice Online Test Question No 6:
Given an account with interest compounded annually, the formula A = P(1 + r)t can be used to calculate the total amount of money, A, in the account after t years, where P is the principal (the amount originally invested) and r is the interest rate (expressed as a decimal). Suppose Valeera invests $5,000 ina savings account that pays 2% interest compounded annually. How much interest willValeera earn in four years? Express your answer to the nearest whole dollar.
Show/Hide Answer Key
Correct Answer: 412
Difficulty: Medium
Category: Passport to Advanced Math / Exponents
Strategic Advice: Even though this question is wordy, if you read carefully you’ll see that you’re given a formula and the values of all the variables except the one you’re looking for. This means you can simply plug in the values and simplify. Before filling in the grid, check that you answered the right question (how much interest was earned, not the total amount in the account).
Getting to the Answer: Jot down the formula A = (1 + r), and then plug in the given values: P = 5,000, r = 0.02, and t = 4, to find that A = 5,000(1 + 0.02) ≈ 5,412.16. This is the total amount in the account after 4 years. The initial amount was $5,000, so subtract to find that Valeera will earn $5,412.16 – $5,000 = $412.16 or, rounded to the nearest whole dollar, $412 in interest.
SAT Math Practice Online Test Question No 7:
When -2x3 – 2x2 + 27x – 30 is divided by 2 – x, what is the remainder?
Show/Hide Answer Key
Correct Answer: 0
Difficulty: Medium
Category: Passport to Advanced Math / Exponents
Strategic Advice: When you are looking for a remainder, long division is definitely the way to go. Just work carefully, particularly with the negative signs.
Getting to the Answer: To reduce the chance of careless errors, start by factoring -1 out of both expressions to remove some of the negative
signs. The math will still work if you omit this step, but you’ll have more negative signs to track.
The remainder is 0.
SAT Math Practice Online Test Question No 8:
A function g satisfies g(5) = 3 and g(3) = 0, and a function h h(3) = –2 and h(0) = 5. What is the value of h(g(3))?
Show/Hide Answer Key
Correct Answer: 5
Difficulty: Medium
Category: Passport to Advanced Math / Functions
Strategic Advice: When asked for the value of a composition of functions, start with the innermost set of parentheses and work outward from there.
Getting to the Answer: The innermost set of parentheses dictates that you should find g(3) first, which the question tells you is equal to 0. Substitute 0 for g(3) in the requested expression to get h(0). The question states that h(0) = 5, so 5 is your final answer.
SAT Math Practice Online Test Question No 9:
If –7 is one solution of the equation y2 + cy – 35 = 0, what is the value of c?
Show/Hide Answer Key
Correct Answer: 2
Difficulty: Easy
Category: Passport to Advanced Math / Quadratics
Strategic Advice: The solution to any equation is a value that satisfies the equation, or in other words, makes the equation true.
Getting to the Answer: Substitute -7 for y and solve for c:
(-7)2 + (-7)c – 35 = 0
49 – 7c – 35 = 0
-7c = -14
c = 2
SAT Math Practice Online Test Question No 10:
1/3(x – 2)2 – 1/2 = 5/2
What is the sum of the roots of the quadratic equation given above?
Show/Hide Answer Key
Correct Answer: 4
Difficulty: Hard
Category: Passport to Advanced Math / Quadratics
Strategic Advice: Roots are the same as solutions to an equation, so you need to solve the equation for x. Don’t let the fractions intimidate you, and don’t jump to the conclusion that you need to find common denominators.
Getting to the Answer: Notice that adding 1/2 to both sides of the equation will actually eliminate two of the fractions. Then, you can multiply both sides of the equation by 3 to eliminate the remaining fraction:
1/2(x – 2)2 – 1/2 = 5/2
1/3(x – 2)2 = 6/2
1/3(x – 2)2 = 3
(x – 2)2 = 9
Both sides of the equation are now perfect squares, so take their square roots. Then solve the resulting equations. Remember, there will be two equations to solve.
(x – 2)2 = 9
√(x – 2) = ±√9
x – 2 = ±3
Now, simplify each equation: x – 2 = -3 yields x = -1, and x – 2 = 3 yields x = 5. The question asks for the sum of the roots, so add -1 + 5 to get 4.