Linear Equations in One Variable
Hi Students, welcome to Amans Maths Blogs (AMB). In this article, CBSE Class 8 Maths Chapter Linear Equations in One Variable is explained. It is the notes of Linear Equations in One Variable chapter which help for the students who study in class 8 of CBSE or any affiliated school.
As we have learnt about the algebraic expressions, which is defined as when constants and variables are combined with mathematical operator to express a mathematical terms known as algebraic expressions.
For example: 4x2y, 7xy + 2xy2, 3x2y2 + 2xy – 5 etc. All are algebraic expressions.
Read : Algebraic Expressions and Identities
Now, an algebraic expression of the form of anxn + an-1xn-1 + an-2xn-2 + … + a3x3 + a2x2 + a1x + a0 is called as polynomial, where n is non-negative integers and an, an-1, an-2, …,a2, a1, a0 all are real numbers known as coefficient of algebraic expression terms.
Read : Integers
For example: the algebraic expression 3x3 + 4x2 – 5x + 8 is a polynomial.
On the basis of degree of polynomials, we have a linear polynomials (ax + b). When this linear polynomial (ax + b) equates to another linear polynomial or a number, then it is known as linear equation in one variable.
In other words, a linear equations is an equation containing algebraic expression in one variable with degree of variable 1.
In the linear equations in one variable, there are two sides known as Left Hand Side (LHS) and Right Hand Side (RHS).
For example: in the standard form of ax + b = 0, LHS = ax + b and RHS = 0.
Now, the main objective to study this chapter linear equations in one variable class 8 is to find the values of variables for which LHS and RHS becomes equal, means LHS = RHS.
Thus, we now understand how to solve a linear equation in one variable.
Solving Linear Equations in One Variable
The solving method of linear equations in one variable depends on the given equations.
Linear Equations of Type 1
In this type, the linear equations contain linear expression in one side and numbers on other side.
It means, the linear equations as ax + b = c.
This equation is solved as below.
ax + b = c ⇒ ax = c – b ⇒ x = (c – b) / a.
For example: solve the linear equation 2x + 15 = 23.
The solution of this equation is as below.
2x + 15 = 23 ⇒ 2x = 23 – 15 ⇒ 2x = 8 ⇒ x = 8/2 ⇒ x = 4.
Thus, the solution of the equation 2x + 15 = 23 is x = 4
Linear Equations of Type 2
In this type, the linear equations contain linear expression in both sides.
It means, the linear equations as ax + b = cx + d.
This equation is solved as below.
ax + b = cx + d ⇒ ax – cx = d – b ⇒ x(a – c) = (d – b) ⇒ x = (d – b) / (a – c).
For example: solve the linear equation 2x – 5 = x + 4.
The solution of this equation is as below.
2x – 5 = x + 4 ⇒ 2x – x = 4 + 5 ⇒ x = 9.
Thus, the solution of the equation 2x – 5 = x + 4 is x = 9.
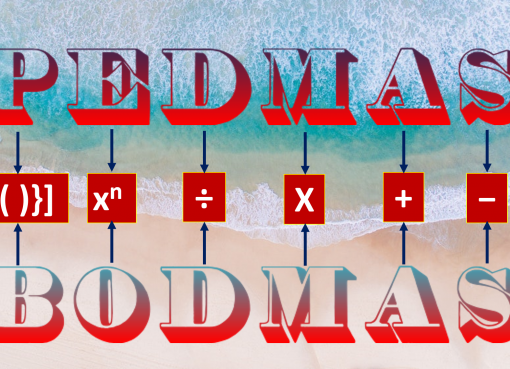
Some linear equations are not simple, then first we need to simplifying it using BODMAS.
Read : Concept of BODMAS or PEDMAS
To understand this, let start with an example.
Suppose we need to solve 25x – 4(4x – 3) = 4(3x – 2) + 7/2.
We solve this equation as below.
25x – 4(4x – 3) = 4(3x – 2) + 7/2
⇒ 25x – 16x + 12 = 12x – 8 + 7/2
⇒ 9x + 12 = 12x – 9/2
⇒ 9x – 12x = -9/2 – 12
⇒ -3x = -33/2
⇒ x = 11/2
Linear Equations of Type 3
In this type, the linear equations are of the form (ax + b) / (cx + d) = p/q
To solve this type of linear equation, first we cross multiplication of the equation and then we solve the same steps as previous example.
(ax + b) / (cx + d) = p/q
⇒ q(ax + b) = p(cx + d)
⇒ qax + bq = pcx + pd
⇒qax – pcx = pd – bq
⇒ x(qa – pc) = pd – bq
⇒ x = (pd – bq) / (qa – pc)
For example: Solve (2x + 3) / (3x – 5) = 7/2
(2x + 3) / (3x – 5) = 7/2
⇒ 2(2x + 3) = 7(3x – 5)
⇒ 4x + 6 = 21x – 35
⇒ 4x – 21x = -35 – 6
⇒ -17x = -41
⇒ x = 41/17
Solving Word Problems Using Linear Equations in One Variable
To solve a word problem using linear equations in one variable ,we follow the following steps:
Step 1 : Read the given word problem carefully and identify that what is given and what is required to find out.
Step 2 : Denote the unknown by any variables like x, y, z, t … etc.
Step 3 : Translate the given problems to the mathematics statements.
Step 4 : Form the linear equation in one variable using the given conditions in the problem.
Step 5 : Solve the linear equation to find the unknown value.
Step 6 : Verify your answer by satisfying given conditions in the word problems.
To understand better, we take an example:
Word Problem:
The difference between two numbers is 70. If the numbers are in ratio 3:5. Then, find the numbers.
Step 1 : We need to find two numbers.
Step 2 : Denote the ratio by x. Thus, two numbers are 3x and 5x
Step 3 : Difference of two numbers = 70
Step 4 : 5x – 3x = 70
Step 5 : 5x – 3x = 70 ⇒ 2x = 70 ⇒ x = 35. Thus, two numbers are 3x = 105 and 5x = 175.
Step 6 : Since 175 – 105 = 70, so our solution is correct.