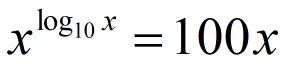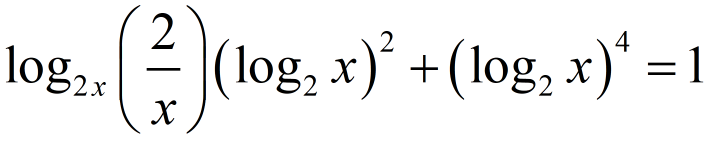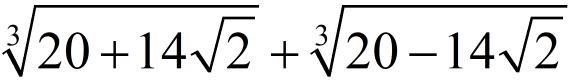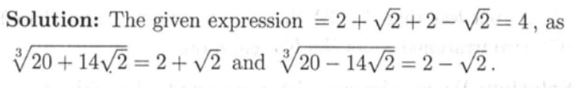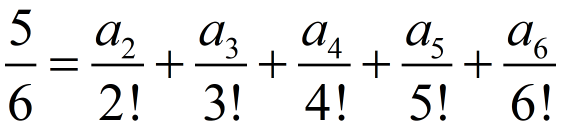NMTC 2010 Question Papers with Solutions Junior Level
NMTC is an acronym for National Mathematics Talent Contest. NMTC is conducted by AMTI (Association of Mathematics Teachers of India) to identify the talent of the students in Mathematics. In this post, NMTC 2010 Question Papers with Solutions Junior Level is published.
Part A: Instruction:
Only One of the choices A, B, C, D is correct. NMTC 2010 Question Papers with Solutions Junior Level For each correct response, you get 1 mark. For each incorrect response, you lose 1/2 mark.
NMTC 2010 Paper For Junior Level Ques No 1:
The numbers which when subtracted from the terms of the ratio a : b makes it equal to c : d is
Options:
A. (ab – cd)/(ab + cd)
B. (bc – ad)/(c + d)
C. (ab + cd)/(c + d)
D. (ab – cd)/(b – c)
Solution:
NMTC 2010 Paper For Junior Level Ques No 2
In a kilometer race, Ram beats Shyam by 25 meters or 5 seconds. The time taken by Ram to complete the race is
Options:
A. 1 minute
B. 5 minute 30 second
C. 3 minute 15 second
D. 4 minute 10 second
Solution:
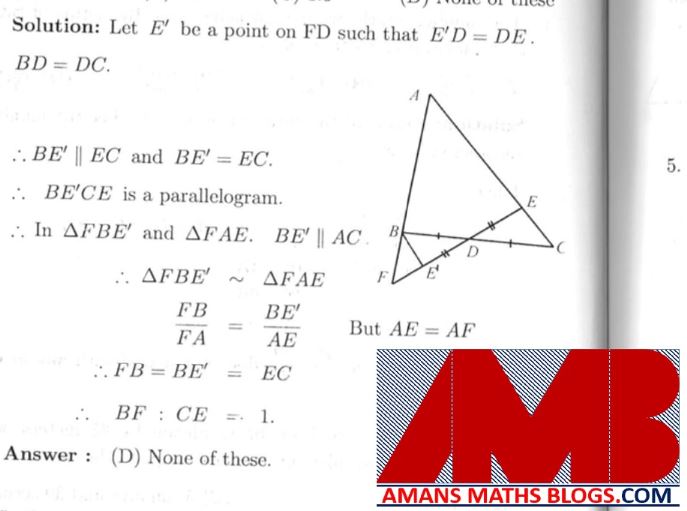
NMTC 2010 Paper For Junior Level Ques No 3
Through D, the mid-point of the side BC of a triangle ABC, a straight line is drawn to meet AC at E and AB produced at F so that AE = AF Then, the ratio of BF : CE is
Options:
A. 1 : 2
B. 2 : 1
C. 1 : 3
D. None of These
Solution:
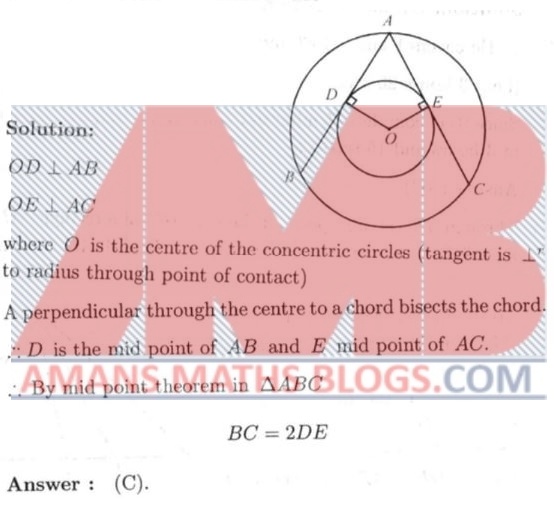
NMTC 2010 Paper For Junior Level Ques No 4
In the bigger of two concentric circles, two chords AB and AC are drawn to touch line is drawn to touch the smaller circle at D and E, then BC is equal to
Options:
A. 3DE
B. 4DE
C. 2DE
D. (3/2)DE
Solution:
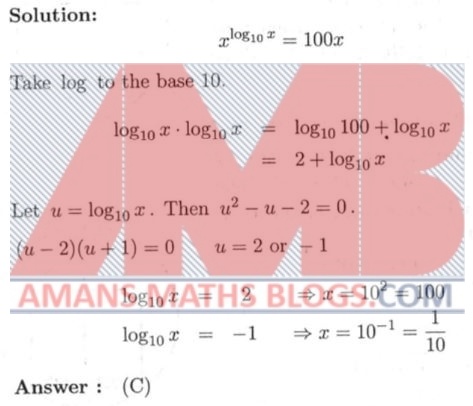
NMTC 2010 Paper For Junior Level Ques No 5
The number of solution of the equation
Options:
A. 0
B. 1
C. 2
D. 3
Solution:
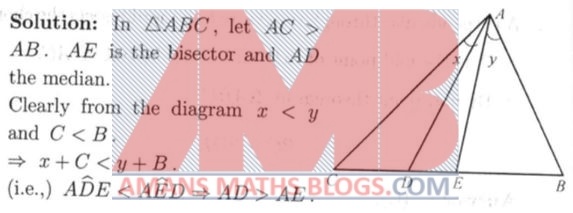
NMTC 2010 Paper For Junior Level Ques No 6
The internal bisector of AE of the angle A of triangle ABC is
Options:
A. Not greater than the median through A for all triangles.
B. Not greater than the median through A for only acute angled triangles.
C. Not greater than the median through A for only obtuse angled triangles.
D. Not less than the median through A for all triangles.
Solution:
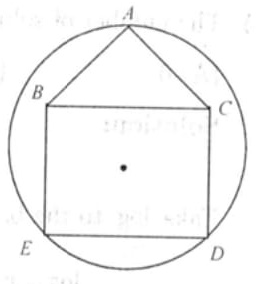
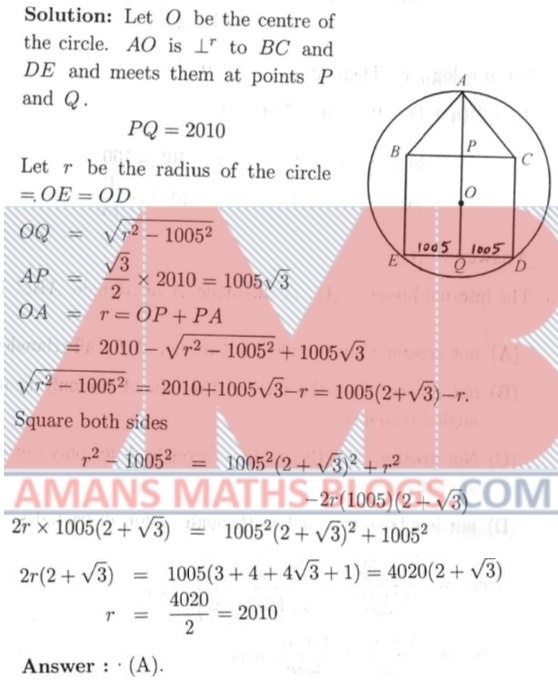
NMTC 2010 Paper For Junior Level Ques No 7
In the adjoining diagram, ABC is an equilateral triangle BCDE is a square. The side of the equilateral triangle is 2010. The radius of the circle is
Options:
A. 2010
B. 4020
C. 6030
D. 8040
Solution:
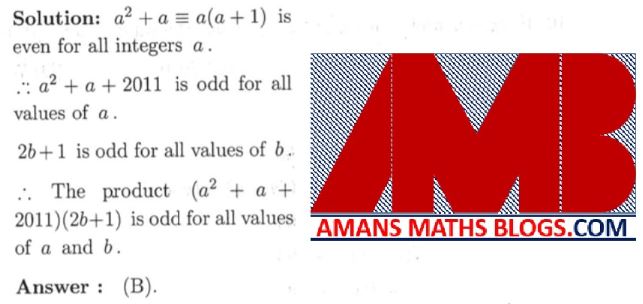
NMTC 2010 Paper For Junior Level Ques No 8
Given a and b are integers the expression (a2 + a + 2011)(2b + 1) is
Options:
A. Odd for exactly 2010 values of a and b.
B. Odd for exactly 2010 values of a and b.
C. Even for exactly one value of a and two values of b.
D. Odd for exactly one value of a and one values of b.
Solution:
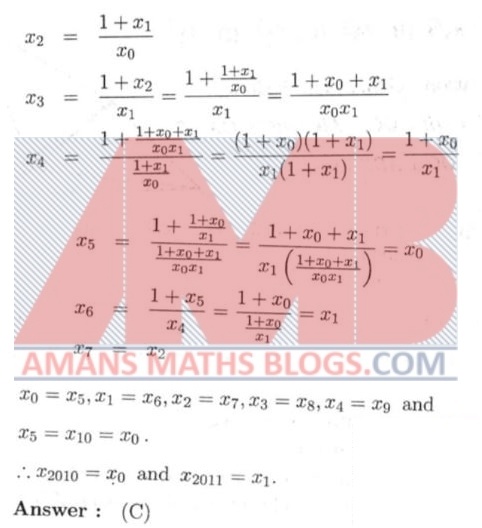
NMTC 2010 Paper For Junior Level Ques No 9
A real sequence of real numbers xn is defined recursively as follows x0, x1, are arbitrary positive real numbers and xn+2 = (1 + xn +1)/xn, n = 0,1,2,3,…. Then the value of x2011 is
Options:
A. 1
B. x0
C. x1
D. x2
Solution:
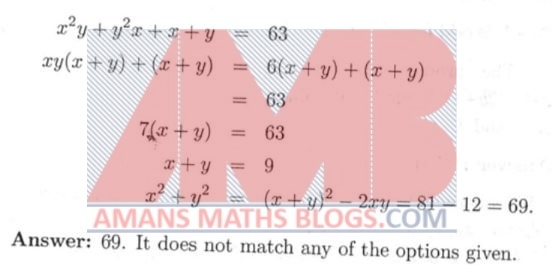
NMTC 2010 Paper For Junior Level Ques No 10
If xy = 6 and x2y + y2x + x + y = 63, then the value of x2 + y2 is
Options:
A. 81
B. 18
C. 2010
D. 78
Solution:
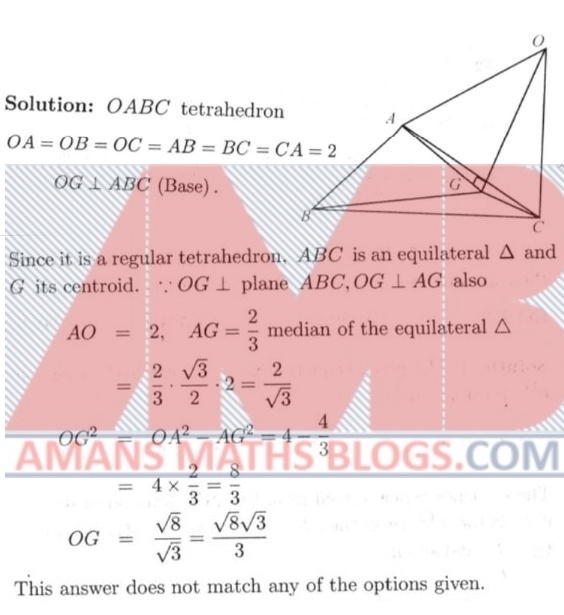
NMTC 2010 Paper For Junior Level Ques No 11
If p is the perpendicular drawn from the vertex of a regular tetrahedron to the opposite face and if each edge is equal to 2 units, the p is
Options:
A.
B. /2
C. /3
D. /5
Solution:
NMTC 2010 Paper For Junior Level Ques No 12
The remainder when the polynomial x + x3 + x9 + x27 + x81 + x243 is divided by x2 – 1.
Options:
A. 6x
B. 2x
C. 3x
D. 1
Solution:
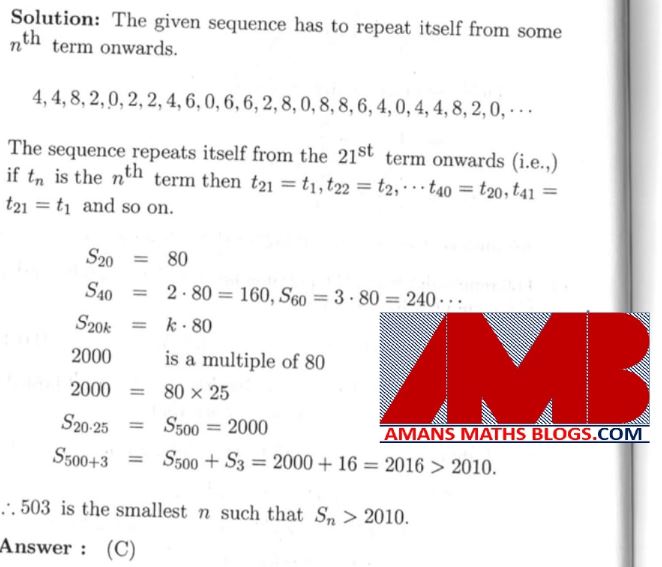
NMTC 2010 Paper For Junior Level Ques No 13
Consider the sequence 4, 4, 8, 2, 0, 2, 4, 6, 0, …where the nth term is the unit place of the sum of the sum of previous two terms for n ≥ 3. If Sn is the sum to n terms of this sequence, then the smallest n for which Sn > 2011 is.
Options:
A. 253
B. 502
C. 503
D. 504
Solution:
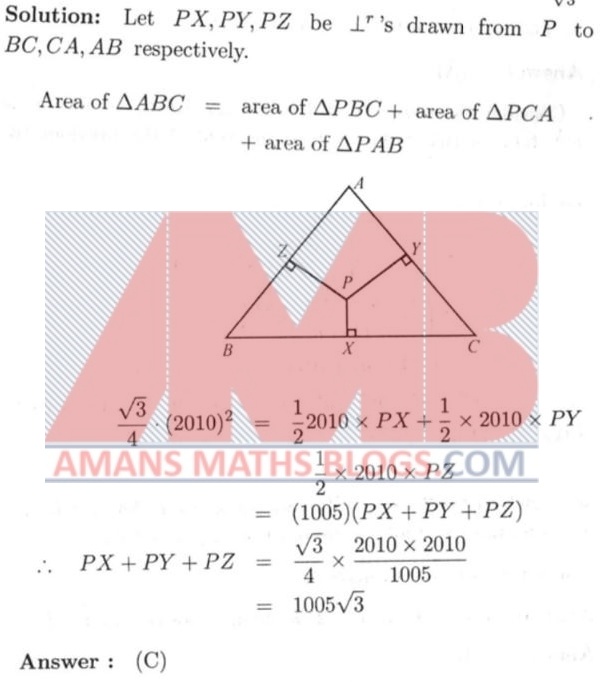
NMTC 2010 Paper For Junior Level Ques No 14
P is the point inside an equilateral triangle of side 2010 units. The sum of the lengths of the perpendiculars drawn from P to the sides is equal to
Options:
A. 2010
B. 2010
C. 1005
D. 2010/
Solution:
NMTC 2010 Paper For Junior Level Ques No 15
The equation
has
Options:
A. A root less than 1
B. Has only root greater than 1
C. Two irrational roots
D. No real roots
Solution:
NMTC 2010 Paper For Junior Level Ques No 16
The value of
is ______.
Solution:
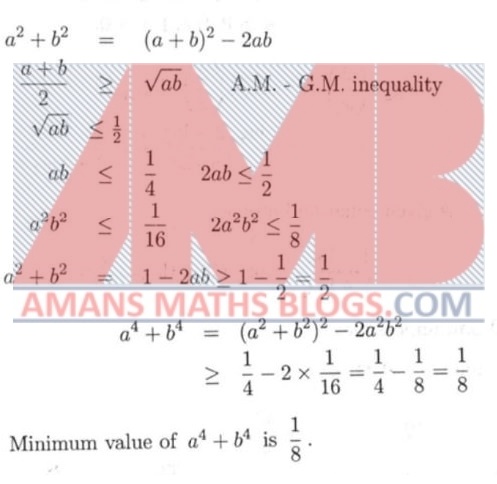
NMTC 2010 Paper For Junior Level Ques No 17
If a, b are positive and a + b = 1 then the minimum value of a4 + b4 is______.
Solution:
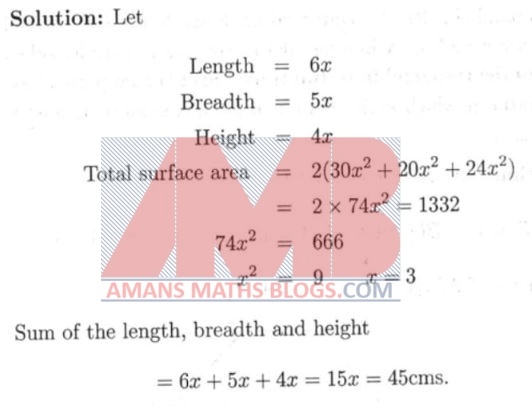
NMTC 2010 Paper For Junior Level Ques No 18
The whole surface area of rectangular block is 1332 cm2. The length, breadth and height are in the ratio of 6:5:4. The sum of the length, breadth and height is _______ cms.
Solution:
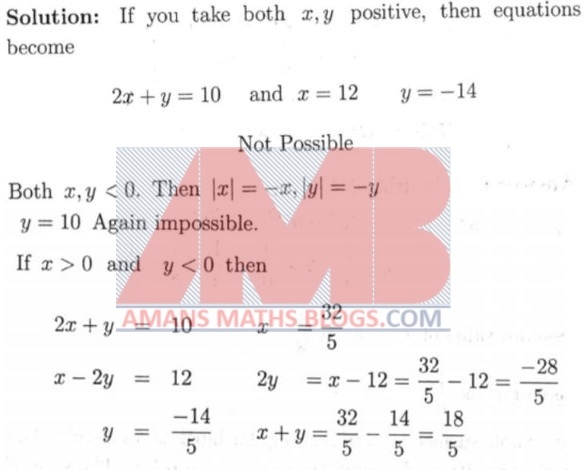
NMTC 2010 Paper For Junior Level Ques No 19
If |x| + x + y = 10 and x + |y| – y = 12, then x + y is _____.
Solution:
NMTC 2010 Paper For Junior Level Ques No 20
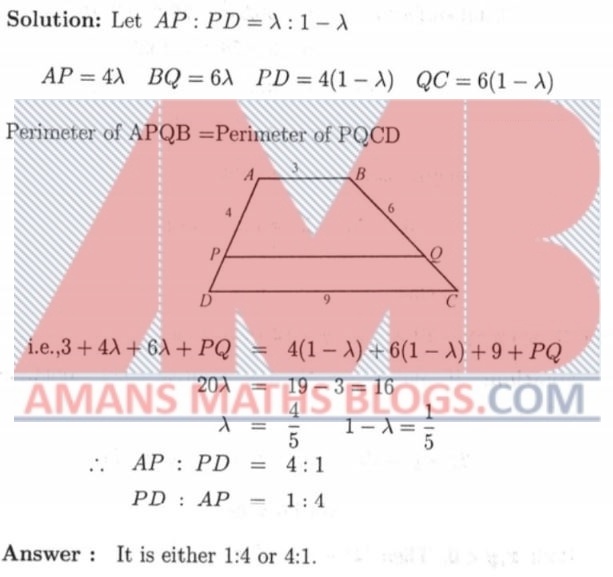
Two parallel sides of a trapezoid are 3 and 9, the non-parallel sides are 4 and 6. A line parallel to the bases (parallel sides) divides the trapezoid into two trapezoids of equal perimeters. The ratio in which each of the non-parallel sides is divided is ______.
Solution:
NMTC 2010 Paper For Junior Level Ques No 21
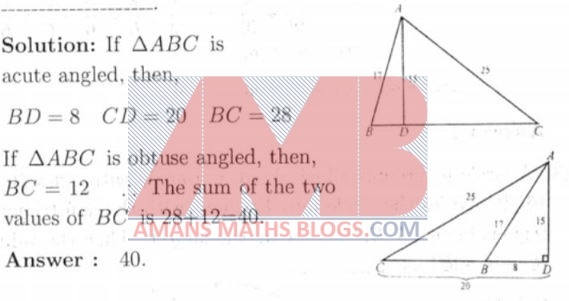
Triangle ABC has AB = 17 and AC = 25 and altitude to BC has length is 15. The sum of possible values of BC is ______.
Solution:
NMTC 2010 Paper For Junior Level Ques No 22
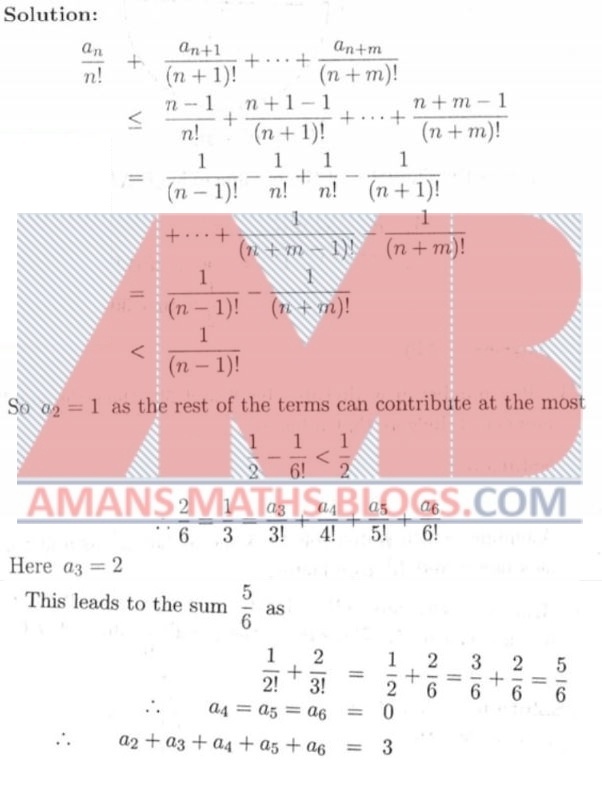
If
where 0 ≤ ai < i, i = 1,2,3,4,5,6. Then, a2 + a3 + a4 + a5 + a6 is ______.
Solution:
NMTC 2010 Paper For Junior Level Ques No 23
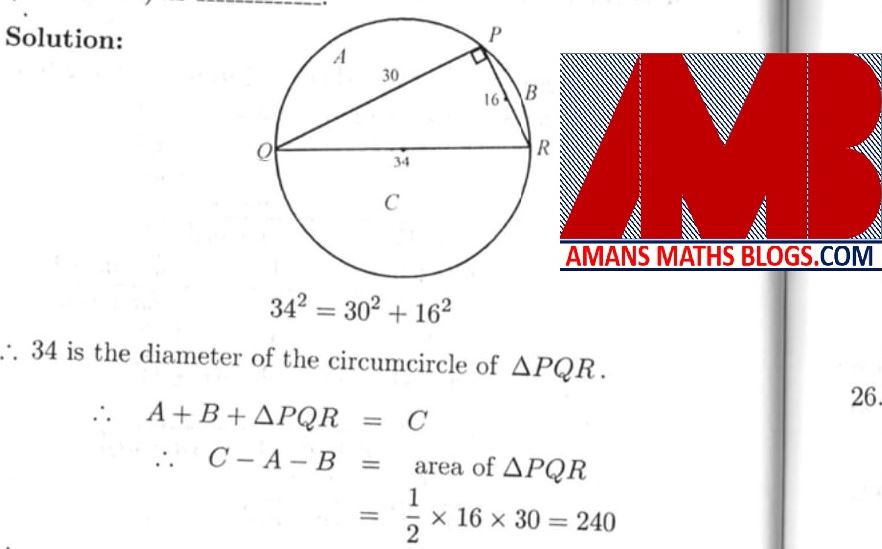
A circle is circumscribed about a triangle with sides 30, 34, 16. It divides the circle into four regions with the non-triangular regions being A, B, C. C being the largest. Then the value of (C – A – B) is ______.
Solution:
NMTC 2010 Paper For Junior Level Ques No 24
If a number n is divisible by 8 and 30, then the smallest number of divisors that n has is ______.
Solution:
NMTC 2010 Paper For Junior Level Ques No 25
Both the quadratic equation x2 – 12x + K = 0 are prime numbers. The sum of all such value of K is _______.
Solution:
NMTC 2010 Paper For Junior Level Ques No 26
In a convex polygon of sides 16 sides the maximum number of angles which can all be equal to 10 degrees is _______.
Solution:
NMTC 2010 Paper For Junior Level Ques No 27
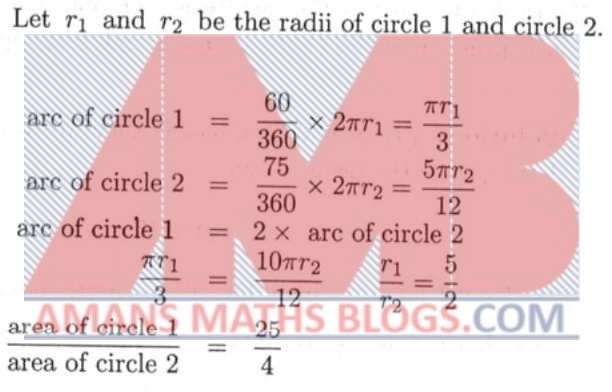
If an arc of circle 1 subtending 60 degree at the centre, has double the length as the arc subtending 75 degrees at the centre in the circle 2, then the ratio of (Area of Circle 1)/ (Area of Circle 2) is ______.
Solution:
NMTC 2010 Paper For Junior Level Ques No 28
A two-digit number is equal to the sum of the product of its digits and the sum of its digits. Then the units place of the number is ______.
Solution:
NMTC 2010 Paper For Junior Level Ques No 29
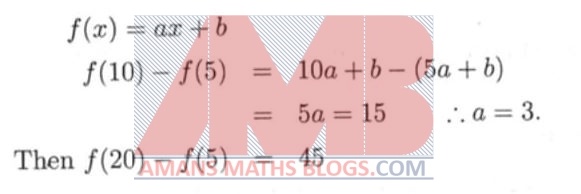
Let f(x) be a polynomial of degree 1. If f(10) – f(5) = 15 and, then f(20) – f(5) is ______.
Solution:
NMTC 2010 Paper For Junior Level Ques No 30
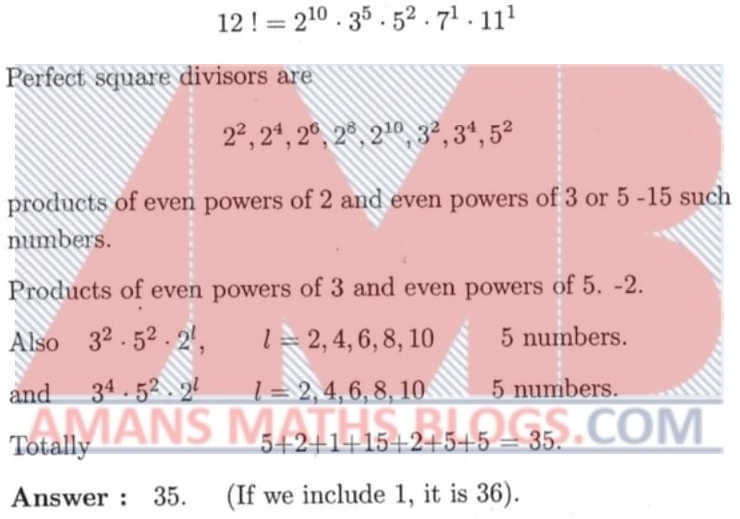
The number of perfect square divisors of the number 12! is _______.
Solution: