Common Core Algebra 1 Unit 2 Equations Formulas Ratio Proportion Chapter Plan
Hi Students, Welcome to Amans Maths Blogs (AMB). In this article, you get the full details of Common Core Algebra 1 Unit 2 Equations Formulas Ratio Proportion Chapter Plan. It means, I describe you about Common Core Algebra 1 Unit 2 Equations Formulas Ratio Proportion, what the chapters and lessons plan. Some definitions and important questions with answers and solutions.
Common Core Algebra 1 Unit 2A Equations and Formulas
Now, there are following chapters in Common Core Algebra 1 Unit 2 Equations Formulas.
Common Core Algebra 1 Unit 2A Chapter 1 Solving Equations by Adding or Subtracting
In this chapter, we learn to solve one step equations in one variable by using addition and subtraction.
As we know that an equation is a mathematical statement that two expression are equal and its solution is a value of variable that makes the equation true.
First, note about the properties of equality.
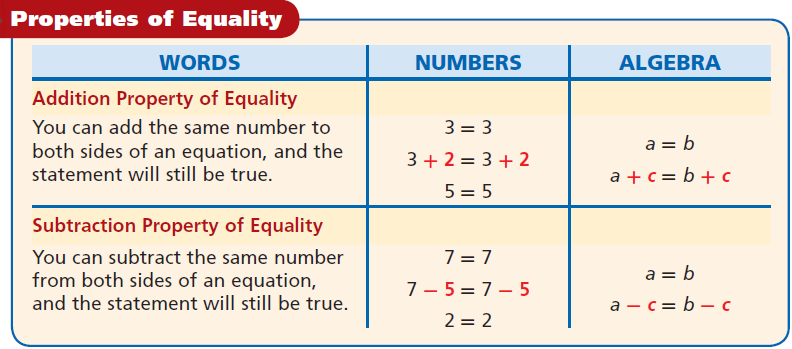
Now, to find the solution of an equation, isolate the variable in one side of the equation by using inverse operation, which undo operation on the variable.
Lets understand it by solving some questions:
Common Core Algebra 1 Unit 2 Chapter 1 Question No : 1
Solve x + 7 = 9
Common Core Algebra 1 Unit 2 Chapter 1 Solution : 1
Given equation is x + 7 = 9.
In LHS, we see that 7 is added to the variable x, so to isolate the variable x in LHS, we subtract 7 from the both sides to undo the addition, this is known as inverse operation.
Thus, we get x + 7 – 7 = 9 – 7 ⇒ x = 2. This x = 2 is the solution of the given equation.
Common Core Algebra 1 Unit 2 Chapter 1 Question No : 2
Solve d + 1/2 = 1
Common Core Algebra 1 Unit 2 Chapter 1 Solution : 2
Given equation is d + 1/2 = 1.
In LHS, we see that 1/2 is added to the variable d, so to isolate the variable d in LHS, we subtract 1/2 from the both sides to undo the addition, this is known as inverse operation.
Thus, we get d + 1/2 – 1/2 = 1 – 1/2 ⇒ d = 1/2. This d = 1/2 is the solution of the given equation.
Common Core Algebra 1 Unit 2 Chapter 1 Question No : 3
Solve x – 5 = 3
Common Core Algebra 1 Unit 2 Chapter 1 Solution : 3
Given equation is x – 5 = 3.
In LHS, we see that 5 is subtracted to the variable x, so to isolate the variable x in LHS, we add 5 to the both sides to undo the subtraction, this is known as inverse operation.
Thus, we get x – 5 + 5 = 3 + 5 ⇒ x = 8. This x = 8 is the solution of the given equation.
Common Core Algebra 1 Unit 2A Chapter 2 Solving Equations by Multiplying or Dividing
In this chapter, we learn to solve one step equations in one variable by using multiplication or division.
Finding the solution of an equation that contains multiplication or division is similar steps as solving in the equation that contains addition or subtraction.
To solve such type of equation, we need to isolate the variable in one side of the equation by using inverse operation, which undo operation on the variable.
Lets understand it by solving some questions:
Common Core Algebra 1 Unit 2 Chapter 2 Question No : 1
Solve 7x = 56
Common Core Algebra 1 Unit 2 Chapter 2 Solution : 1
Given equation is 7x = 56.
In LHS, we see that 7 is multiplied to the variable x, so to isolate the variable x in LHS, we divide 7 to both sides to undo the multiplication, this is known as inverse operation.
Thus, we get 7x / 7 = 56 / 7 ⇒ x = 8. This x = 8 is the solution of the given equation.
Common Core Algebra 1 Unit 2 Chapter 2 Question No : 2
Solve x/6 = 102
Common Core Algebra 1 Unit 2 Chapter 2 Solution : 2
Given equation is w/6 = 102.
In LHS, we see that 6 is divided to the variable w, so to isolate the variable w in LHS, we multiply 6 to both sides to undo the division, this is known as inverse operation.
Thus, we get w/6 = 102 x 6 ⇒ w = 612. This w = 612 is the solution of the given equation.
Common Core Algebra 1 Unit 2 Chapter 2 Question No : 3
Write an equation to represent the relation “Five time a number is 45”, and then solve the equation.
Common Core Algebra 1 Unit 2 Chapter 2 Solution : 3
Let the number is x. Then, we have equation as 5x = 45.
In LHS, we see that 5 is multiplied to the variable x, so to isolate the variable x in LHS, we divide 5 to both sides to undo the multiplication, this is known as inverse operation.
Thus, we get 5x / 5 = 45 / 5 ⇒ x = 9. This x = 9 is the solution of the given equation.
Common Core Algebra 1 Unit 2A Chapter 3 Solving Two Steps & Multi Steps Equation
In this chapter, we learn to solve two or More steps equations in one variable.
To understand better, let an example of equation as
This equation contains multiplication and addition. Equation that contain more than one operation require more than one steps to solve this equation. Identify the order of operations in the equation and order in which they are applied to the variables. Then use inverse operation and work backward to undo them one at a time. Thus, we do the steps as below.
Therefore, we get
⇒ 3.95c + 19.95 – 19.95 = 63.40 – 19.95
⇒ 3.95c = 43.45
⇒ 3.95c / 3.95 = 43.45/3.95
⇒ c = 11
Lets solve some questions:
Common Core Algebra 1 Unit 2 Chapter 3 Question No : 1
If 2x + 4 = -24, then find x.
Common Core Algebra 1 Unit 2 Chapter 3 Solution : 1
2x + 4 = -24
⇒ 2x + 4 – 4 = -2.4 – 4
⇒ 2x = -6.4
⇒ 2x / 2 = -6.4/2
⇒ x = -3.2
Common Core Algebra 1 Unit 2 Chapter 3 Question No : 2
If x/6 + 4 = 15, then find x.
Common Core Algebra 1 Unit 2 Chapter 3 Solution : 2
x/6 + 4 = 15
⇒ x/6 + 4 – 4 = 15 – 4
⇒ x/6 = 11
⇒ x/6 * 6 = 11 * 6
⇒ x = 66
Common Core Algebra 1 Unit 2 Chapter 3 Question No : 3
If 3(x – 4) = 48, then find x.
Common Core Algebra 1 Unit 2 Chapter 3 Solution : 3
3(x – 4) = 48
⇒ 3(x – 4) / 3 = 48 / 3
⇒ x – 4 = 16
⇒ x – 4 + 4 = 16 + 4
⇒ x = 20
Common Core Algebra 1 Unit 2A Chapter 4 Solving Equations with Variables on Both Sides
In this chapter, we learn to solve the equations containing variables on both sides.
To understand better, start with an example.
We need to find the value of x in the equation
7k = 4k + 15 …(1)
To solve such types of equations, first of all we need to collect all variables on one side.
We need to subtract 4k from both sides of the equation (1)
7k – 4k = 4k + 15 – 4k
⇒ 3k = 15
Since k is multiplied by 3, divided both sides by 3 to undo the multiplication.
⇒ k = 5
Lets solve some questions:
Common Core Algebra 1 Unit 2 Chapter 4 Question No : 1
If 4b + 2 = -3b, then find b.
Common Core Algebra 1 Unit 2 Chapter 4 Solution : 1
4b + 2 = -3b
⇒ 4b + 2 + 3b = -3b + 3b
⇒ 7b + 2 = 0
⇒ 7b + 2 – 2 = 0 – 2
⇒ 7b = – 2
⇒ 7b / 7 = – 2 / 7
⇒ b = – 2 / 7
Common Core Algebra 1 Unit 2 Chapter 4 Question No : 2
If 4y + 7 – y = 10 + 2y, then find y.
Common Core Algebra 1 Unit 2 Chapter 4 Solution : 2
4y + 7 – y = 10 + 2y
⇒ 3y + 7 = 10 + 2y
⇒ 3y + 7 – 2y = 10 + 2y – 2y
⇒ y + 7 = 10
⇒ y + 7 – 7 = 10 – 7
⇒ y = 3
Common Core Algebra 1 Unit 2A Chapter 5 Solving For Variables
In this chapter, we will learn how to solve the equations for variables in terms of other variables.
A formula is an equation that states a rule for a relationship among quantities. The formula is a type of literal equation with two or more variables
To solve a equation for variable in terms of other variables, we do the following steps.
Step 1: Locate the variables you are asked to solve for in the equation.
Step 2: Identify the operations on this variables and the order in which they are applied.
Step 3: Use inverse operations and isolate the variables.
Lets solve some questions:
Common Core Algebra 1 Unit 2 Chapter 5 Question No : 1
The formula for a Fahrenheit temperature in terms of degree Celsius is F = (9/5)C + 32. Solve for C.
Common Core Algebra 1 Unit 2 Chapter 5 Solution : 1
Given equation is F = (9/5)C + 32.
⇒ F – 32 = (9/5)C + 32 – 32
⇒ F – 32 = (9/5)C
⇒ (F – 32) x (5/9) = (9/5)C x (5/9)
⇒ (F – 32) x (5/9) = C
Thus, C = (5/9)(F – 32)
Common Core Algebra 1 Unit 2 Chapter 5 Question No : 2
Solve for t in the equation 5 – b = 2t.
Common Core Algebra 1 Unit 2 Chapter 5 Solution : 2
Given equation is 5 – b = 2t.
⇒ (5 – b) / 2 = 2t / 2
⇒ (5 – b)/2 = t
Thus, t = (5 – b)/2
Common Core Algebra 1 Unit 2 Chapter 5 Question No : 3
Solve for n in the equation m/n = p – 6.
Common Core Algebra 1 Unit 2 Chapter 5 Solution : 3
Given equation is m/n = p – 6.
⇒ (m/n) x n = (p – 6) x n
⇒ m = (p – 6) x n
⇒ m/(p – 6) = n
Thus, n = m/(p – 6)
Common Core Algebra 1 Unit 2A Chapter 6 Solving Absolute Value Equations
In this chapter, we will learn how to solve the equations containing the absolute values.
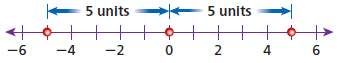
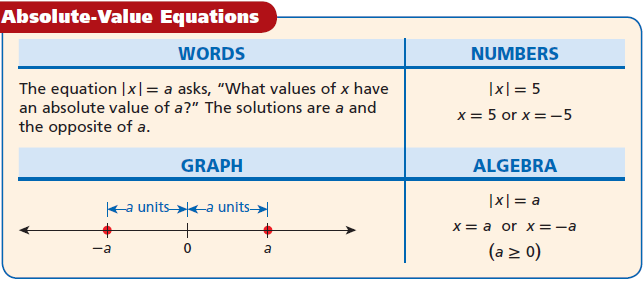
As we know that an absolute value of a number is the number’s distance from zero on a number line. Absolute of a number x is represented as |x|, which is read as MOD of x.
For example: |5| = 5 and |-5| = 5. It means, for any nonzero value, there are exactly two numbers with that absolute.
Now, we need to ask a question that what the absolute value of x is 5, it means we need to solve for x in the question |x| = 5.
To solve the absolute value equation, perform inverse operations to isolate the absolute value expression on one side of the equation. For this, we need to must consider two cases.
Lets solve some questions:
Common Core Algebra 1 Unit 2 Chapter 6 Question No : 1
Solve for x in the equation |x| = 6.
Common Core Algebra 1 Unit 2 Chapter 6 Solution : 1
Given absolute value equation is |x| = 6.
⇒ x = 6 or -6
Common Core Algebra 1 Unit 2 Chapter 6 Question No : 2
Solve for x in the equation |x – 3| – 6 = 2.
Common Core Algebra 1 Unit 2 Chapter 6 Solution : 2
Given absolute value equation is |x – 3| – 6 = 2.
⇒ |x – 3| -6 + 6 = 2 + 6
⇒ |x – 3| = 8
⇒ x – 3 = 8 or -8
⇒ x – 3 + 3 = (8 + 3) or (-8 + 3)
⇒ x = 11 or -5
Common Core Algebra 1 Unit 2 Chapter 6 Question No : 3
Solve for x in the equation 3|x| – 12 = 18.
Common Core Algebra 1 Unit 2 Chapter 6 Solution : 3
Given absolute value equation is 3|x| – 12 = 18.
⇒ 3|x| – 12 + 12 = 18 + 12
⇒ 3|x| = 30
⇒ 3|x| / 3 = 30 / 3
⇒ |x| = 10
⇒ x = 10 or -10
Common Core Algebra 1 Unit 2B Chapter 7 Rates Ratio & Proportion
In this chapter, we will learn about the ratio and proportions.
A ratio is a comparison of two quantities by division. The ratio of a to b can be written a : b or a/b, where b is non-zero. For example: the ration of 1 and 12 is 1 : 12 or 1/12.
Ratios that give same comparison are called as Equivalent ratios. For example: 1/12 and 2/24 are equivalent ratios.
A ratio is differ from fraction. A ratio can be positive or negative but a fraction is always positive.
Read More: 8 Types of Fractions
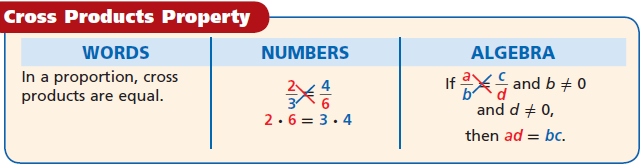
Now, a statement that two ratios are equivalent is called as Proportion. For example: 1/12 = 2/24 is called Proportion. In proportion, a/b = c/d, the products a.d and b.c are called cross product.
A rate is a ratio of two quantities with different units and second means denominator quantity of 1 unit. For example: 10km/5hour = 2km/1hour. This is the rate.
Lets solve some questions:
Common Core Algebra 1 Unit 2 Chapter 7 Question No : 1
Solve the proportion: 3/z = 1/8.
Common Core Algebra 1 Unit 2 Chapter 7 Solution : 1
Given proportion is 3/z = 1/8.
⇒ z x 1 = 3 x 8
⇒ z = 24
Common Core Algebra 1 Unit 2 Chapter 7 Question No : 2
Solve the proportion: 9/2 = 5/(k + 1).
Common Core Algebra 1 Unit 2 Chapter 7 Solution : 2
Given proportion is 9/2 = 5/(k + 1).
⇒ 9 x (k + 1) = 2 x 5
⇒ 9(k + 1) = 10
⇒ (k + 1) = 10/9
⇒ (k + 1 – 1) = 10/9 – 1
⇒ k = 1/9
Common Core Algebra 1 Unit 2 Chapter 7 Question No : 3
Solve the proportion: 3/(d + 3) = 4/(d + 12).
Common Core Algebra 1 Unit 2 Chapter 7 Solution : 3
Given proportion is 3/(d + 3) = 4/(d + 12).
⇒ 3(d + 12) = 4(d + 3)
⇒ 3d + 36 = 4d + 12
⇒ 3d + 36 – 3d = 4d + 12 – 3d
⇒ 36 = d + 12
⇒ 36 – 12 = d + 12 – 12
⇒ 24 = d
Thus, d = 24
Common Core Algebra 1 Unit 2B Chapter 8 Applications of Proportions
In this chapter, we will learn about the applications of proportions.
The proportion can be used to solve the questions involving figures, specially similar figure.
A similar figures have exactly the same shape but not necessarily the same shape.
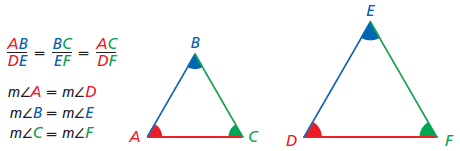
Corresponding sides of two figures are in the same relative position and corresponding angles are equal.
For example: if two triangles ABC and DEF are similar, then
Lets solve some questions:
Common Core Algebra 1 Unit 2 Chapter 8 Question No : 1
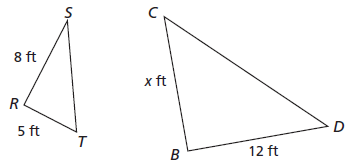
In the figure below, if the triangles RST and BCD are similar, then find the value of x.
Common Core Algebra 1 Unit 2 Chapter 8 Solution : 1
Since ∆RST ~ ∆BCD, then RS/BC = RT/BD = ST/CD
⇒ RS/BC = RT/BD (Using only first two fractions)
⇒ 8/x = 5/12
⇒ x = (8 * 12) / 5 = 19.2
Common Core Algebra 1 Unit 2 Chapter 8 Question No : 2
In the figure below, if the hexagons FGHJKL and MNPQRS are similar, then find the value of x.
Common Core Algebra 1 Unit 2 Chapter 8 Solution : 2
Since FGHJKL ~ MNPQRS, then NP/GH = RQ/KJ
⇒ 6/4 = x/2
⇒ x = (6 * 2) / 4 = 3
Common Core Algebra 1 Unit 2 Chapter 8 Question No : 3
In the figure below, if the triangles ABC and DEF are similar, then find the value of x.
Common Core Algebra 1 Unit 2 Chapter 8 Solution : 31
Since ∆ABC ~ ∆DEF, then AB/DE = AC/DF = BC/EF
⇒ AB/DE = AC/DF (Using only first two fractions)
⇒ 5/4 = 7/x
⇒ 5x = 4*7
⇒ x = 28 / 5 = 5.6
Common Core Algebra 1 Unit 2B Chapter 9 Percents
In this chapter, we will learn about the percentage.
A PERCENT is a ratio that compares a number to 100. A percentage is represented as % sign.
To convert a ratio into percent, multiply the ratio with 100 and then write the simplification with % sign.
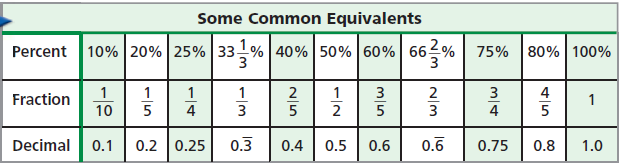
For example: 1/4 = (1/4) x 100 = 25%
And, to convert a percentage into a ratio, write the percent with a denominator with of 100, then simplify it.
We can also change it into decimal by dividing the percent by 100, as below.
Lets solve some questions:
Common Core Algebra 1 Unit 2 Chapter 9 Question No : 1
Find 20% of 60.
Common Core Algebra 1 Unit 2 Chapter 9 Solution : 1
Let x represent 20% of 60, then
⇒ x = 20% of 60
⇒ x = (0.2) 60
⇒ x = 12
Thus, 20% of 60 is 12.
Common Core Algebra 1 Unit 2 Chapter 9 Question No : 2
Find 210% of 8.
Common Core Algebra 1 Unit 2 Chapter 9 Solution : 2
Let x represent 210% of 8, then
⇒ x = 210% of 8
⇒ x = (2.1) 8
⇒ x = 16.8
Thus, 210% of 8 is 16.8.
Common Core Algebra 1 Unit 2 Chapter 9 Question No : 3
Find 4% of 36.
Common Core Algebra 1 Unit 2 Chapter 9 Solution : 3
Let x represent 4% of 36, then
⇒ x = 4% of 36
⇒ x = (0.04) 36
⇒ x = 1.44
Thus, 4% of 36 is 1.44.
Common Core Algebra 1 Unit 2B Chapter 10 Applications of Percents
In this chapter, we will learn about the applications of percents.
Percents can be used in finding Simple Interest and Sales Tax.
Interest is the amount of money charged for borrowing money, or the amount of money when saving or investing money. Principle is the amount borrowed or invested. Simple Interest is the interest paid only on the principle.
A tip is an amount of money added to a bill for service. It is usually a percent of the bill before sales tax is added. Sales Tax is a percent of an item’s cost.
Lets solve some questions:
Common Core Algebra 1 Unit 2 Chapter 10 Question No : 1
A telemarketers earns $350 per week plus a 12 % commission on sales. Find her total pay for a week in which her sales are $940.
Common Core Algebra 1 Unit 2 Chapter 10 Solution : 1
Total Pay = Earning Per Week + Commission
⇒ Total Pay = 350 + (12% of 350)
⇒ Total Pay = 350 + (0.12 x 350)
⇒ Total Pay = 350 + 42
⇒ Total Pay = $392
Common Core Algebra 1 Unit 2 Chapter 10 Question No : 2
Find the simple interest earned after 2 years on an investment of $3000 at 4.5% interest earned annually.
Common Core Algebra 1 Unit 2 Chapter 10 Solution : 2
I = PRT
⇒ I = (3000) x (4.5%) x (2)
⇒ I = (3000) x (4.5/100) x (2)
⇒ I = (3000) x (45/1000) x (2)
⇒ I = (3) x (45) x (2)
⇒ I = $270
Common Core Algebra 1 Unit 2 Chapter 10 Question No : 3
Estimate a 15% tip on a check for $21.98
Common Core Algebra 1 Unit 2 Chapter 10 Solution : 3
First, we round up $21.98 to $22.
Now Tip is about to 15% of $22.
⇒ Required tip = 15% of $22
= (10% of $22) + (5% of $22)
= $2.2 + $1.1 = $3.3
Common Core Algebra 1 Unit 2B Chapter 11 Percentage Increase & Decrease
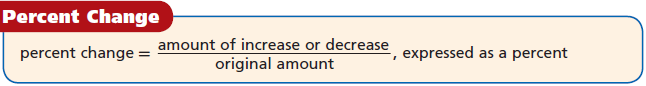
In this chapter, we will learn about the percentage increase or decrease. A percent change is an increase or decrease given as a percent of the original amount. Percent increase describes an amount that has grown and percent decrease describes an amount that has been reduced.
The main applications of percentage increase or decrease are in DISCOUNT and MARKUP.
A discount is an amount by which an original price is reduced. A markup is an amount by which is an amount a wholesale cost is increased.
Lets solve some questions:
Common Core Algebra 1 Unit 2 Chapter 11 Question No : 1
Find the percent change, if a number is changed from 200 to 110.
Common Core Algebra 1 Unit 2 Chapter 11 Solution : 1
= (200 – 110) / 200
= 90/200
= 90/200 x 100
= 45%
Since the number change from 200 to 110 is decrease, so a change from 200 to 110 is a 45% decrease.
Common Core Algebra 1 Unit 2 Chapter 11 Question No : 2
Find the percent change, if a number is changed from 25 to 30.
Common Core Algebra 1 Unit 2 Chapter 11 Solution : 2
= (30 – 25) / 25
= 5/25
= 1/5
= 1/5 x 100
= 20%
Since the number change from 25 to 30 is increase, so a change from 25 to 30 is a 20% increase.