NMTC 2016 Question Papers with Solutions Inter Level
NMTC is an acronym for National Mathematics Talent Contest. NMTC is conducted by AMTI (Association of Mathematics Teachers of India) to identify the talent of the students in Mathematics. In this post, NMTC 2016 Question Papers with Solutions Inter Level is published.
Part A: Instruction:
Only One of the choices A, B, C, D is correct. For each correct response, you get 1 mark. For each incorrect response, you lose 1/2 mark.
NMTC 2016 Paper For Inter Level Ques No 1:
P is a point on AL, the altitude of the triangle ABC through A. If angle PBA = 20 Degree, angle PBC = 40 Degree and angle PCB = 30 Degree, then angle PCA equals
Options:
A. 20 degree
B. 10 degree
C. 15 degree
D. 18 degree
Solution:
NMTC 2016 Paper For Inter Level Ques No 2:
ABCDE is a regular pentagon. The area of the star shaped region ACEBDA is 1 Square cm. AC and BE meet at P and BD and CE meet at Q as shown in the figure. The area of APQD in square cms is
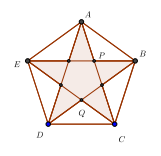
Options:
A. 1/4
B. 1/3
C. 1/2
D. 3/4
Solution:
NMTC 2016 Paper For Inter Level Ques No 3:
The number of integer pairs (x, y) which satisfy the equation x3 = y3 + 2y2 + 1 is
Options:
A. 0
B. 1
C. 2
D. 3
Solution:
NMTC 2016 Paper For Inter Level Ques No 4:
Let (x) = x4 + x3 + x2 + x + 1. The reminder when (x5) is divided by (x) is
Options:
A. 5
B. 6
C. 7
D. 4
Solution:
NMTC 2016 Paper For Inter Level Ques No 5:
The number of positive integers a, b, c such that a2 + b2 + c2 = a2b2 is
Options:
A. 1
B. 2
C. 5
D. None of these
Solution:
NMTC 2016 Paper For Inter Level Ques No 6:
The number of triples (x, y, z) of real numbers such that 3x2 + y2 + z2 = 2x(y + z) is
Options:
A. 1
B. 2
C. 3
D. 5
Solution:
NMTC 2016 Paper For Inter Level Ques No 7:
The number of real roots of the equation x4 – 4x = 1 is
Options:
A. 1
B. 2
C. 0
D. 4
Solution:
NMTC 2016 Paper For Inter Level Ques No 8:
Given a set of r points in the plane so that no three are collinear, by a closed polygon we mean the polygon obtained by connecting them by r line segments as shown in the examples below (here r = 5 ). There are 10 points on a plane no three of which are collinear. The number of 5 sided closed polygons whose vertices are among these 10 points is
Options:
A. 6048
B. 1507
C. 3024
D. 10000
Solution:
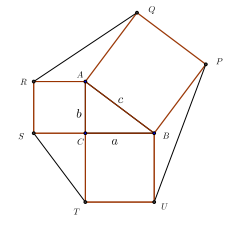
NMTC 2016 Paper For Inter Level Ques No 9:
In the adjacent figure ABC is a right angled triangle. Square are described externally on its sides and the outer vertices of these squares are joined as shown. If the lengths of the sides AB, BC, CA are respectively c, a, b the area of the hexagon PQRSTU is
Options:
A. 2(a2 + ab + b2)
B. (a2 + ab + b2)
C. 3(a2 + ab + b2)
D. (1/2)(a2 + ab + b2)
Solution:
NMTC 2016 Paper For Inter Level Ques No 10:
ABCD is a square. From B, D lines are drawn to meet at P inside the square such that ∠ADP = 25◦ and ∠ABP = 20◦ . Then ∠BP C is
Options:
A. 2(a2 + ab + b2)
B. (a2 + ab + b2)
C. 3(a2 + ab + b2)
D. (1/2)(a2 + ab + b2)
Solution:
NMTC 2016 Paper For Inter Level Ques No 11:
If p, q are positive odd integers such that (1 + 3 + 5 + · · · + p) + (1 + 3 + 5 + · · · + q) = 1 + 3 + · · · + 19 then p + q is
Options:
A. A Prime Number
B. Divisible by 13
C. Odd Number
D. None of these
Solution:
NMTC 2016 Paper For Inter Level Ques No 12:
The number 220 − 1 is divisible by
Options:
A. 11 and 41
B. 11 and 21
C. 41 and 61
D. 11 and 61
Solution:
NMTC 2016 Paper For Inter Level Ques No 13:
Five points O, A, B, C, D are taken in order on a straight line such that OA = a, OB = b, OC = c and OD = d . P is a point on the line between B and C . If AP : P D = BP : P C , then OP is
Options:
A. (ac – bd) / (a – b + c – d)
B. (ac + bd) / (a – b + c – d)
C. (ad – bc) / (a – b + c – d)
D. None of these
Solution:
NMTC 2016 Paper For Inter Level Ques No 14:
The side AB of an equilateral triangle AB is produced to D such that BD = 2AB. The point F is the foot of the perpendicular from D on CB produced. ∠FAC =
Options:
A. 70 degree
B. 75 degree
C. 80 degree
D. 90 degree
Solution:
NMTC 2016 Paper For Inter Level Ques No 15:
For the simultaneous equations x2 + 2xy + y2 − x − y = 6, x − 2y = 3
Options:
A. There is a solution (x, y) such that both x, y are irrational.
B. There are two sets of solutions (x, y) such that x, y are integers.
C. Sum of all solutions is 1.
D. Product of all solutions is 5/3.
Solution:
Part B: Instruction:
Write the correct answer in the space provided in the responsive sheet. For each correct response, you get 1 mark. For each incorrect response, you lose 1/4 mark. In this post, NMTC 2016 Question Papers with Solutions Inter Level is published.
NMTC 2016 Paper For Inter Level Ques No 16:
The number of right angled triangles with integer side lengths and such that the product of the lengths of the legs (non-hypotenuse sides) equals three times the perimeter of the triangle is ____.
Solution:
NMTC 2016 Paper For Inter Level Ques No 17:
α, β, γ, δ are the roots of the equation x4 − ax3 + ax2 + bx + c = 0 where a, b, c are real numbers. The smallest possible value of α2 + β2 + γ2 + δ2 is _____.
Solution:
NMTC 2016 Paper For Inter Level Ques No 18:
Two circles with centers P and Q and radii 3 and 4 respectively touch each other externally. AB, CD are direct common tangents touching the smaller circle at A, C and the bigger circle at C, D. The area of the concave hexagon APCDQB is _____.
Solution:
NMTC 2016 Paper For Inter Level Ques No 19:
If a, b are the lengths of unequal diagonals of a regular heptagon (regular polygon with 7 sides) with side c , then 1/a + 1/b in terms of c is ____.
Solution:
NMTC 2016 Paper For Inter Level Ques No 20:
The number of real roots of the equation 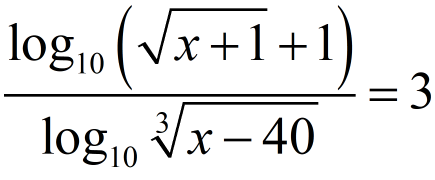
Solution:
NMTC 2016 Paper For Inter Level Ques No 21:
x, y, z are non-zero real numbers such that x2 + y2 +z2 = 1 and x(1/y + 1/z) + y(1/z + 1/x) + z(1/x + 1/y) + 3 = 0. The number of possible values of x + y + z is _____.
Solution:
NMTC 2016 Paper For Inter Level Ques No 22:
The minimum value of integer n such that among any n integers we can always find three integers whose sum is divisible by 3 is ____.
Solution:
NMTC 2016 Paper For Inter Level Ques No 23:
The number of integers n for which n4 − 51n2 + 50 is negative is _____.
Solution:
NMTC 2016 Paper For Inter Level Ques No 24:
In a triangle ABC, the lengths of the sides are consecutive integers and the median drawn from A is perpendicular to the bisector of angle B . The largest side of the triangle has length _____.
Solution:
NMTC 2016 Paper For Inter Level Ques No 25:
a, b, c, d, e are real numbers such that a + 4b + 9c + 16d + 25e = 1, 4a + 9b + 16c + 25d + 36e = 8, 9a + 16b + 25c + 36d + 49e = 23. Then the value of a + b + c + d + e is ____.
Solution:
NMTC 2016 Paper For Inter Level Ques No 26:
In a triangle ABC, the altitude, angle bisector and the median from C divide the angle C into four equal angles. The measure of the least angle of the triangle is ____.
Solution:
NMTC 2016 Paper For Inter Level Ques No 27:
AB is a chord of a circle with center O. AB is produced to C such that BC = OA. CO is produced to E. The value of (angle AOE) / (angle ACE) is _____.
Solution:
NMTC 2016 Paper For Inter Level Ques No 28:
The number of two digit numbers that are less than the sum of the squares of their digits by 11 and exceed twice the product of their digits by 5 is ____.
Solution:
NMTC 2016 Paper For Inter Level Ques No 29:
ABD is a circle whose Centre is C. The circle circumscribing ABC cuts DA or DA produced at E. Then the triangle BDE is a ____ triangle.
Solution:
NMTC 2016 Paper For Inter Level Ques No 30:
The number of 4-digit numbers N such that (a) No digit of N is 9, (b) N is the square of an integer, (c) When each digit of N is increased by 1, the resulting number is also the square of an integer, is_____.
Solution:
Answer Keys:
1> A
2> C
3> D
4> A
5> D
6> A
7> B
8> C
9> A
10> A
11> B
12> A
13> A
14> D
15> C
16> 3
17> -1
18> 28
19> 1/c
20> 1
21> 3
22> 5
23> 12
24> 4
25> 4
26> 22.5 degree
27> 3
28> 2
29> Isosceles
30> 1