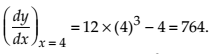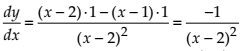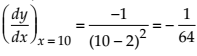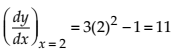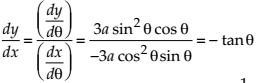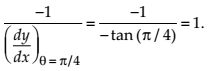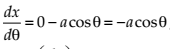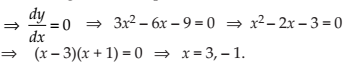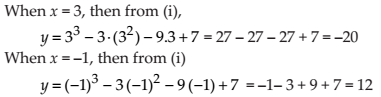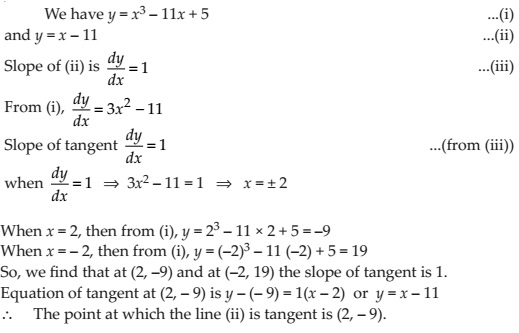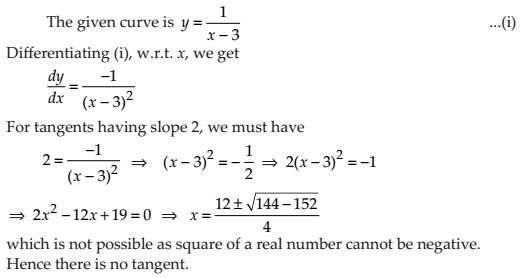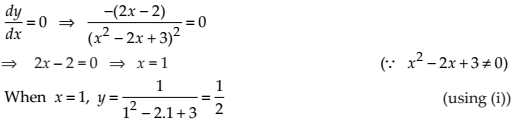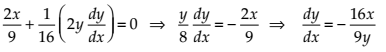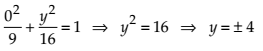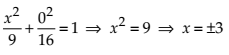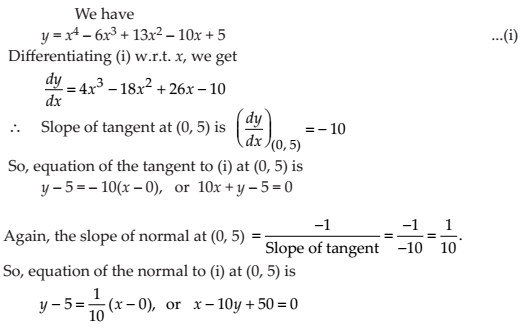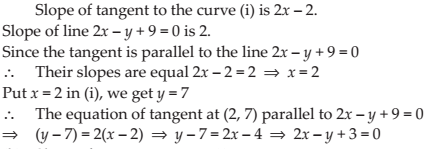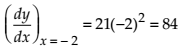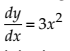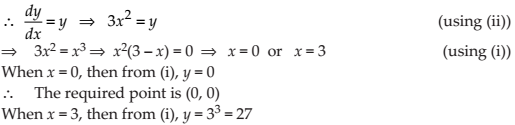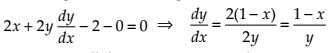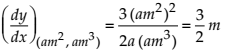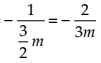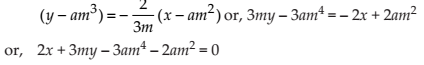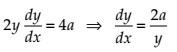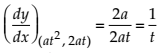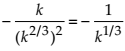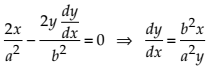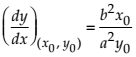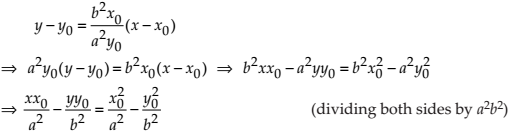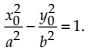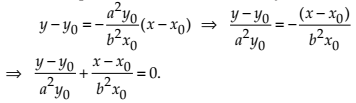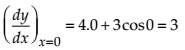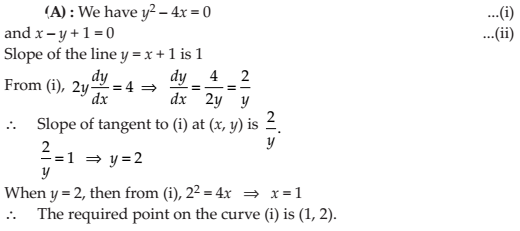NCERT Solutions for Class 12 Maths Application of Derivatives
Hi Students, Welcome to Amans Maths Blogs (AMB). In this post, you will get the NCERT Solutions for Class 12 Maths Application of Derivative Exercise 6.3.
As we know that all the schools affiliated from CBSE follow the NCERT books for all subjects. You can check the CBSE NCERT Syllabus. Thus, NCERT Solutions helps the students to solve the exercise questions as given in NCERT Books.
NCERT Solutions for Class 12 Maths are not only the solutions of Maths exercise but it builds your foundation of other important subjects. Getting knowledge of depth concept of CBSE Class 12th Maths like Algebra, Calculus, Trigonometry, Coordinate Geometry help you to understand the concept of Physics and Physical Chemistry.
The PDF books of NCERT Solutions for Class 12 are the first step towards the learning and understanding the each sections of Maths, Physics, Chemistry, Biology as it all help in engineering medical entrance exams. To solve it, you just need to click on download links of NCERT solutions for class 12.
CBSE Class 12th is an important school class in your life as you take some serious decision about your career. And out of all subjects, Maths is an important and core subjects. So CBSE NCERT Solutions for Class 12th Maths is major role in your exam preparation as it has detailed chapter wise solutions for all exercise. This NCERT Solutions can be downloaded in PDF file. The downloading link is given at last.
NCERT Solutions for class 12 is highly recommended by the experienced teacher for students who are going to appear in CBSE Class 12 and JEE Mains and Advanced and NEET level exams. Here You will get NCERT Solutions for Class 12 Maths Application of Derivative Exercise 6.2 of all questions given in NCERT textbooks of class 12 in details with step by step process.
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 1.
Find the slope of the tangent to the curve y = 3x4 – 4x at x = 4.
NCERT Solutions:
We are given that y = f(x) = 3x4 – 4x.
On differentiating f(x) with respect to x, we get
Thus, the slope of tangent to the curve at x = 4 is
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 2.
Find the slope of the tangent to the curve y = (x – 1)/(x – 2), x ≠ 2 at x = 10.
NCERT Solutions:
We are given that y = f(x) = (x – 1)/(x – 2).
On differentiating f(x) with respect to x, we get
Thus, the slope of tangent to the curve at x = 10 is
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 3.
Find the slope of the tangent to curve y = x3 – x + 1 at the point whose x-coordinate is 2.
NCERT Solutions:
We are given that y = f(x) = x3 – x + 1.
On differentiating f(x) with respect to x, we get
Thus, the slope of tangent to the curve at x = 2 is
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 4.
Find the slope of the tangent to the curve y = x3 –3x + 2 at the point whose x-coordinate is 3
NCERT Solutions:
We are given that y = f(x) = x3 – 3x + 2.
On differentiating f(x) with respect to x, we get
Thus, the slope of tangent to the curve at x = 3 is
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 5.
Find the slope of the normal to the curve x = acos3 θ, y = asin3 θ at θ = π/4.
NCERT Solutions:
We are given that x = acos3 θ, y = asin3 θ.
On differentiating x = acos3 θ with respect to θ, we get
On differentiating y = asin3 θ with respect to θ, we get
Thus, we have
Thus, the slope of normal to the curve at θ = π/4 is
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 6.
Find the slope of the normal to the curve x = 1− asinθ, y = bcos2 θ at θ = π/2.
NCERT Solutions:
We are given that x = 1− asinθ, y = bcos2 θ.
On differentiating x = 1− asinθ with respect to θ, we get
On differentiating y = bcos2 θ with respect to θ, we get
Thus, we have
Thus, the slope of normal to the curve at θ = π/2 is
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 7.
Find points at which the tangent to the curve y = x3 – 3x2 – 9x + 7 is parallel to the x-axis.
NCERT Solutions:
We are given that y = f(x) = x3 – 3x2 – 9x + 7. …(1)
On differentiating f(x) with respect to x, we get
Since the tangent is parallel to x-axis, then
Thus, the required points are (3, -20) and (-1, 12).
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 8.
Find a point on the curve y = (x – 2)2 at which the tangent is parallel to the chord joining the points (2, 0) and (4, 4).
NCERT Solutions:
We are given that y = f(x) = (x – 2)2. …(1)
On differentiating f(x) with respect to x, we get
Since the slope of chord joining two points (2, 0) and (4, 4) is
Then, we have
From the equation (1), we get y = (x – 2)2 = 1.
Thus, the required point is (3, 1).
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 9.
Find the point on the curve y = x3 – 11x + 5 at which the tangent is y = x – 11.
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 10.
Find the equation of all lines having slope – 1 that are tangents to the curve y = 1/(x – 1), x ≠ 1.
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 11.
Find the equation of all lines having slope 2 that are tangents to the curve y = 1/(x – 3), x ≠ 3.
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 12.
Find the equation of all lines having slope 0 that are tangents to the curve y = (x2 – 2x + 3).
NCERT Solutions:
We are given that y = f(x) = x2 – 2x + 3. …(1)
On differentiating f(x) with respect to x, we get
Since the slope of the tangent is 0, then
Thus, the tangent to the given curve at (1, 1/2) has its slope = 0 and the equation of the tangent is
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 13.
Find points on the curve x2 /9 + y2 /16 = 1 at which the tangents are (i) parallel to x-axis (ii) parallel to y-axis.
NCERT Solutions:
We are given that x2/9 + y2/16 = 1. …(1)
On differentiating equation (1) with respect to x, we get
(i) Since the tangent is parallel to x-axis, then
From the equation (1), we have
Thus, the required points are (0, 4) and (0, -4).
(i) Since the tangent is parallel to y-axis, then
From the equation (1), we have
Thus, the required points are (3, 0) and (-3, 0).
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 14.
Find the equations of the tangent and normal to the given curves at the indicated points:
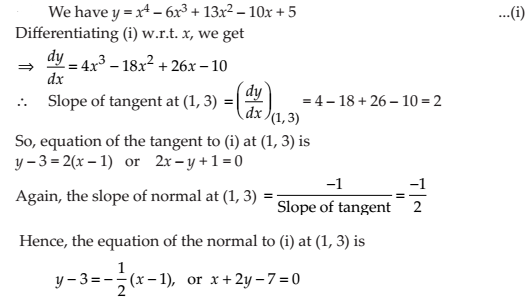
(i) y = x4 – 6x3 + 13x2 – 10x + 5 at (0, 5)
NCERT Solutions:
(ii) y = x4 – 6x3 + 13x2 – 10x + 5 at (1, 3)
NCERT Solutions:
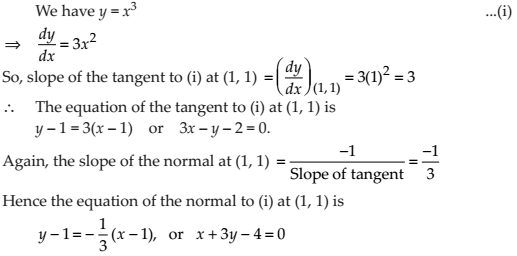
(iii) y = x3 at (1, 1)
NCERT Solutions:
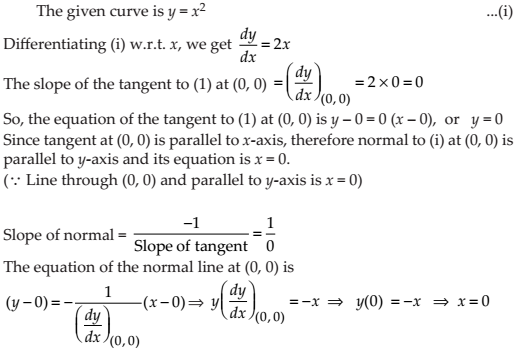
(iv) y = x2 at (0, 0)
NCERT Solutions:
(v) x = cos t, y = sin t at t = π/4.
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 15.
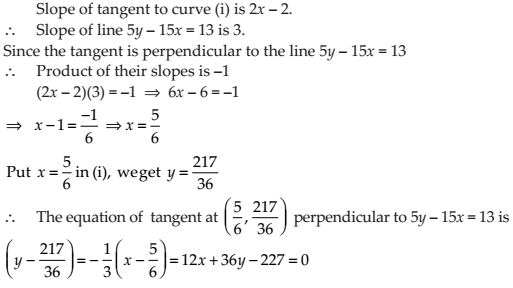
Find the equation of the tangent line to the curve y = x2 – 2x +7 which is
(a) parallel to the line 2x – y + 9 = 0
(b) perpendicular to the line 5y – 15x = 13.
NCERT Solutions:
We are given that 2x – y + 9 = 0. …(1)
On differentiating equation (1) with respect to x, we get
(a)
(b)
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 16.
Show that the tangents to the curve y = 7x3 + 11 at the points where x = 2 and x = – 2 are parallel.
NCERT Solutions:
We are given that y = f(x) = 7x3 + 11.
On differentiating f(x) with respect to x, we get
Thus, the slope of tangent to the curve at x = 2 is
And, the slope of tangent to the curve at x = -2 is
Thus, the tangents to the given curve at the points where x = 2 and x = – 2 are parallel.
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 17.
Find the points on the curve y = x3 at which the slope of the tangent is equal to the y-coordinate of the point.
NCERT Solutions:
We are given that y = f(x) = x3. …(1)
On differentiating f(x) with respect to x, we get
Since the slope of tangent is equal to the y-coordinate of the point.
Thus, the required points are (0, 0) and (3, 7).
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 18.
For the curve y = 4x3 – 2x5, find all the points at which the tangent passes through the origin.
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 19.
Find the points on the curve x2 + y2 – 2x – 3 = 0 at which the tangents are parallel to the x-axis.
NCERT Solutions:
We are given that x2 + y2 – 2x – 3 = 0. …(1)
On differentiating (1) with respect to x, we get
Since the tangents are parallel to x-axis, then the slope of the tangent is 0, then
From the equation (1), we get
Thus, the required points are (1, 2) and (1, -2).
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 20.
Find the equation of the normal at the point (am2,am3) for the curve ay2 = x3.
NCERT Solutions:
We are given that ay2 = x2. …(1)
On differentiating (1) with respect to x, we get
Thus, the slope of tangent at (am2, am3) to the given curve.
Now, the slope of the normal is
Thus, the equation of the normal to the given curve is
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 21.
Find the equation of the normals to the curve y = x3 + 2x + 6 which are parallel to the line x + 14y + 4 = 0.
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 22.
Find the equations of the tangent and normal to the parabola y2 = 4ax at the point (at2, 2at).
NCERT Solutions:
We are given that y2 = 4ax.
On differentiating with respect to x, we get
Thus, the slope of tangent to the curve at (at2, 2at) is
Thus, the equation of the tangent to the given curve is
Now, the slope of the normal is
Thus, the equation of the normal to the given curve is
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 23.
Prove that the curves x = y2 and xy = k cut at right angles if 8k2 = 1.
NCERT Solutions:
We are given that
x = y2 … (1)
xy = k …(2)
On solving the equations (1) and (2), we get
and
Thus, the given curves intersect at
Now, on differentiating (1) with respect to x, we get
Thus, the slope of tangent to the curve at (k2/3, k1/3) is m1 =
Now, on differentiating (2) with respect to x, we get
Thus, the slope of tangent to the curve at (k2/3, k1/3) is m2 =
Since both curves intersect at right angle, the product of the slopes of the tangents m1m2 = -1.
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 24.
Find the equations of the tangent and normal to the hyperbola x2/a2 – y2/b2 = 1 at the point (x0, y0).
NCERT Solutions:
We are given that x2/a2 – y2/b2 = 1.
On differentiating with respect to x, we get
Thus, the slope of tangent to the curve at (x0, y0) is
Thus, the equation of the tangent to the given curve at (x0, y0) is
Since
Therefore, the equation of the tangent to the given curve at (x0, y0) is
Now, the slope of the normal at (x0, y0) is
Thus, the equation of the normal to the given curve is
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 25.
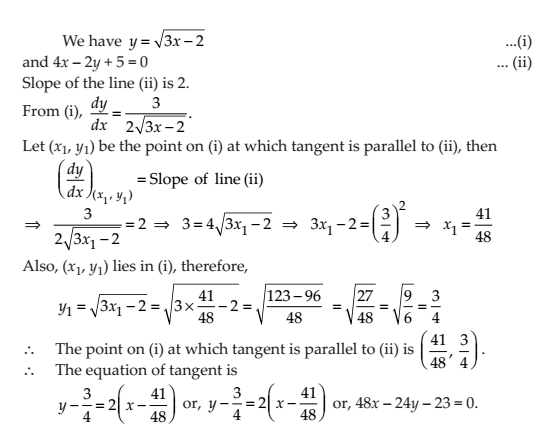
Find the equation of the tangent to the curve which is parallel to the line 4x − 2y + 5 = 0
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 25.
The slope of the normal to the curve y = 2x2 + 3 sin x at x = 0 is (A) 3 (B) 1/3 (C) -3 (D) -1/3
NCERT Solutions:
We are given that y = 2x2 + 3sin x.
On differentiating with respect to x, we get
Thus, the slope of tangent to the curve at x = 0 is
Now, the slope of the normal at x = 0 is
NCERT Solutions for Class 12 Maths Application of Derivatives Exercise 6.3: Ques No 26.
The slope of the normal to the curve y = 2x2 + 3 sin x at x = 0 is (A) 3 (B) 1/3 (C) -3 (D) -1/3
NCERT Solutions: