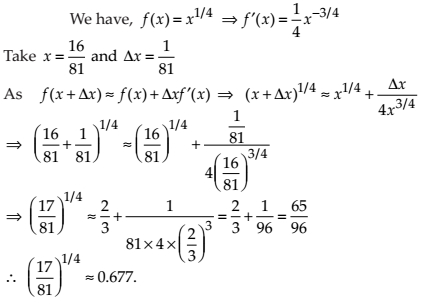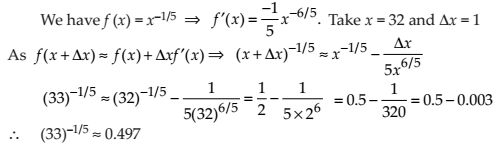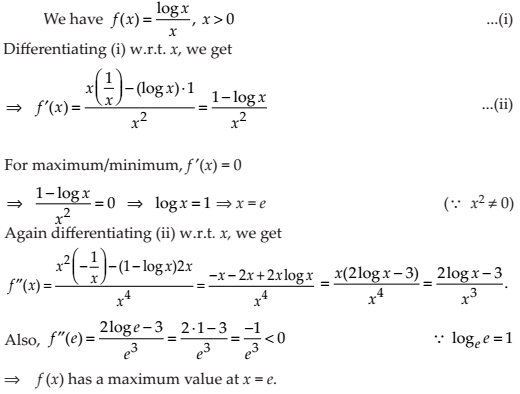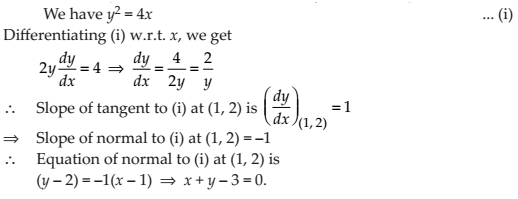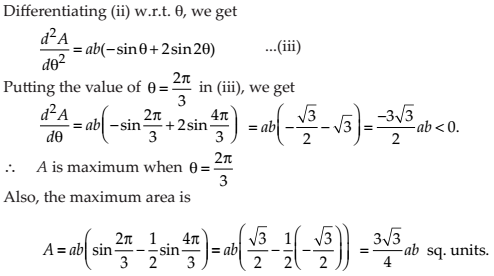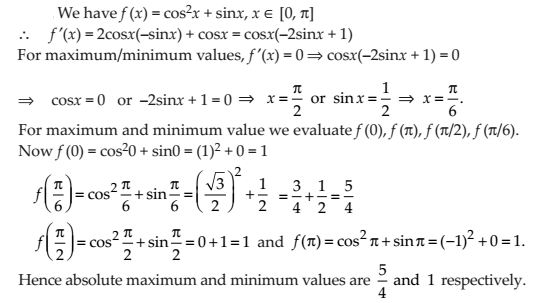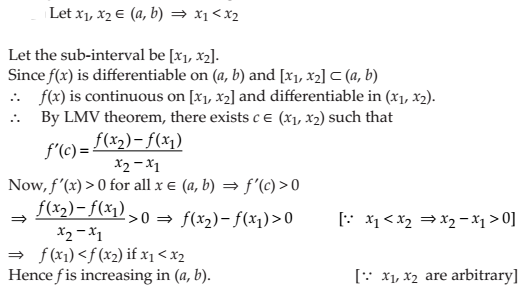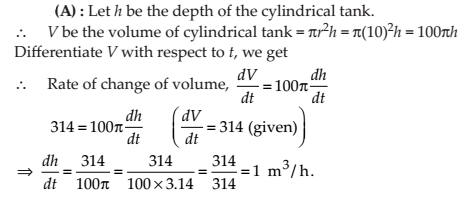NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise
Hi Students, Welcome to Amans Maths Blogs (AMB). In this post, you will get the NCERT Solutions for Class 12 Maths Application of Derivative Miscellaneous Exercise.
CBSE Class 12th is an important school class in your life as you take some serious decision about your career. And out of all subjects, Maths is an important and core subjects. So CBSE NCERT Solutions for Class 12th Maths is major role in your exam preparation as it has detailed chapter wise solutions for all exercise. This NCERT Solutions can be downloaded in PDF file. The downloading link is given at last.
NCERT Solutions for class 12 is highly recommended by the experienced teacher for students who are going to appear in CBSE Class 12 and JEE Mains and Advanced and NEET level exams. Here You will get NCERT Solutions for Class 12 Maths Application of Derivative Exercise 6.2 of all questions given in NCERT textbooks of class 12 in details with step by step process.
NCERT Solutions for Class 12 Maths are not only the solutions of Maths exercise but it builds your foundation of other important subjects. Getting knowledge of depth concept of CBSE Class 12th Maths like Algebra, Calculus, Trigonometry, Coordinate Geometry help you to understand the concept of Physics and Physical Chemistry.
As we know that all the schools affiliated from CBSE follow the NCERT books for all subjects. You can check the CBSE NCERT Syllabus. Thus, NCERT Solutions helps the students to solve the exercise questions as given in NCERT Books.
The PDF books of NCERT Solutions for Class 12 are the first step towards the learning and understanding the each sections of Maths, Physics, Chemistry, Biology as it all help in engineering medical entrance exams. To solve it, you just need to click on download links of NCERT solutions for class 12.
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 1.
(a)
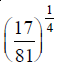
NCERT Solutions:
(b)
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 2.
Show that the function given by f (x) = (log x)/x has maximum at x = e.
NCERT Solutions:
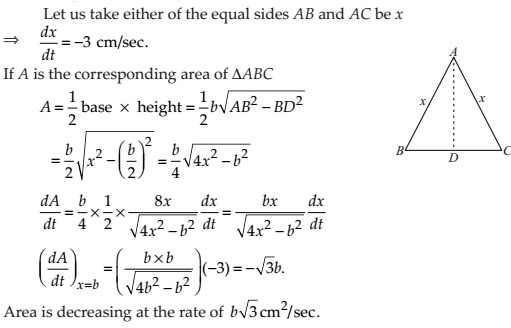
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 3.
The two equal sides of an isosceles triangle with fixed base b are decreasing at the rate of 3 cm per second. How fast is the area decreasing when the two equal sides are equal to the base ?
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 4.
Find the equation of the normal to curve y2 = 4x which passes through the point (1, 2).
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 5.
Show that the normal at any point θ to the curve x = a cosθ + a θ sin θ, y = a sinθ – aθ cosθ
is at a constant distance from the origin.
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 6.
Find the intervals in which the function f given by is f(x) = (4sin x – 2x – x cosx)/(2 + cos x) (i) increasing (ii) decreasing.
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 7.
Find the intervals in which the function f given by f (x) = x3 + 1/x3, x ≠ 0 is (i) increasing (ii) decreasing.
NCERT Solutions:
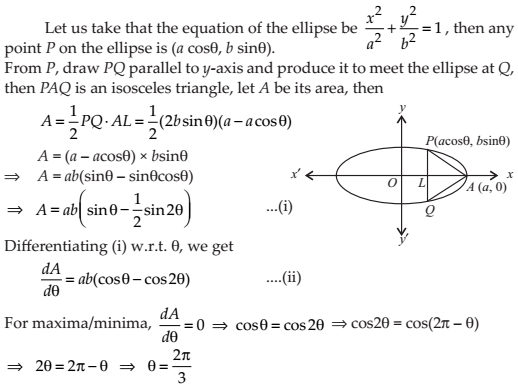
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 8.
Find the maximum area of an isosceles triangle inscribed in the ellipse x2/a2 + y2/b2 = 1 with its vertex at one end of the major axis.
NCERT Solutions:
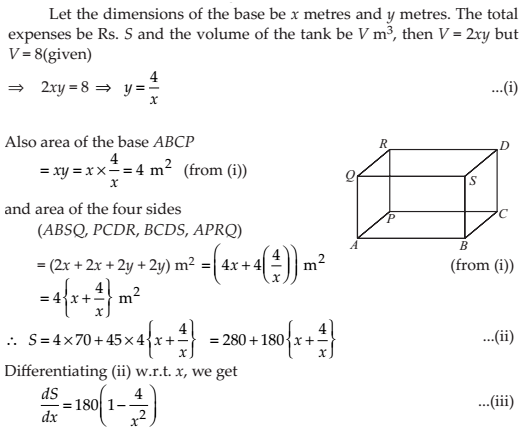
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 9.
A tank with rectangular base and rectangular sides, open at the top is to be constructed so that its depth is 2 m and volume is 8 m3. If building of tank costs Rs 70 per sq metres for the base and Rs 45 per square metre for sides. What is the cost of least expensive tank?
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 10.
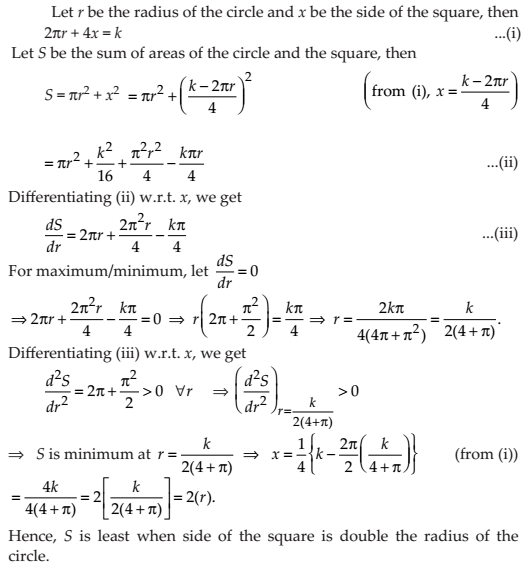
The sum of the perimeter of a circle and square is k, where k is some constant. Prove that the sum of their areas is least when the side of square is double the radius of the circle
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 11.
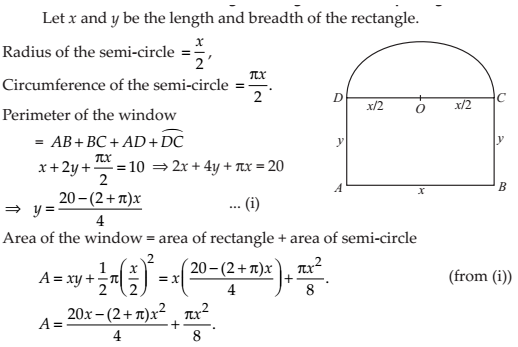
A window is in the form of a rectangle surmounted by a semicircular opening. The total perimeter of the window is 10 m. Find the dimensions of the window to admit maximum light through the whole opening.
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 12.
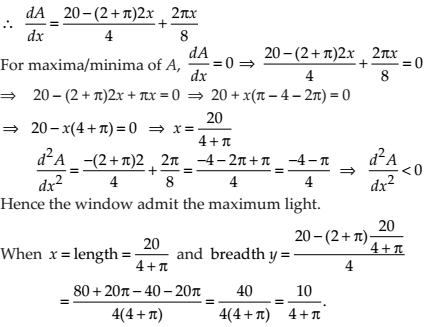
A point on the hypotenuse of a triangle is at distance a and b from the sides of the triangle. Show that the maximum length of the hypotenuse is .
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 13.
Find the points at which the function f given by f (x) = (x – 2)4 (x + 1)3 has (i) local maxima (ii) local minima (iii) point of inflexion.
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 14.
Find the absolute maximum and minimum values of the function f given by f (x) = cos2 x + sin x, x ∈ [0, π].
NCERT Solutions:
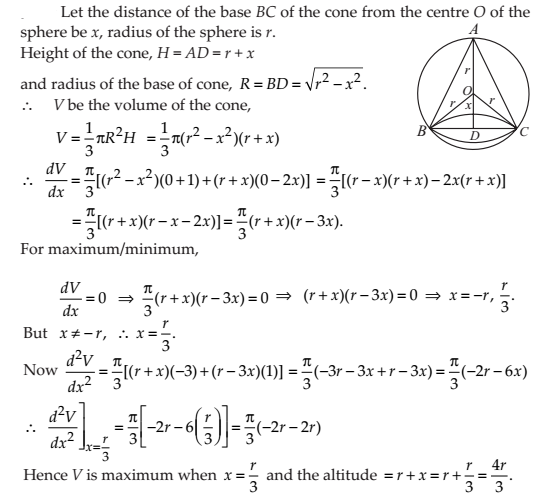
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 15.
Show that the altitude of the right circular cone of maximum volume that can be inscribed in a sphere of radius r is 4r/3.
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 16.
Let f be a function defined on [a, b] such that f ′(x) > 0, for all x ∈ (a, b). Then, prove that f is an increasing function on (a, b).
NCERT Solutions:
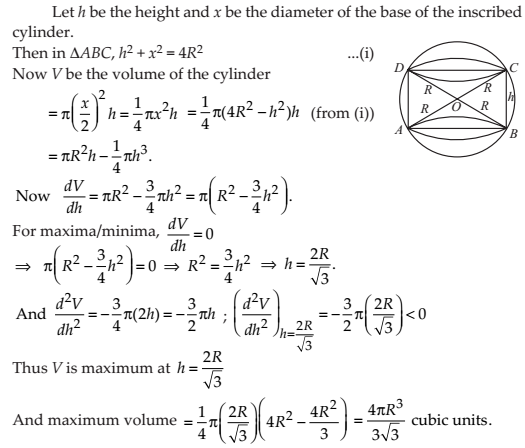
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 17.
Show that the height of the cylinder of maximum volume that can be inscribed in a sphere of radius R is 2R/√3 . Also find the maximum volume.
NCERT Solutions:
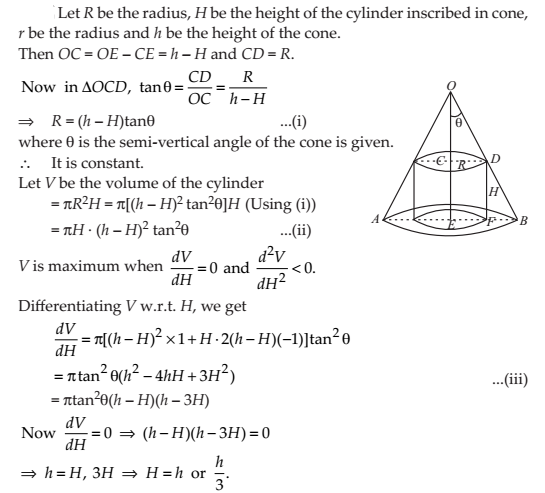
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 18.
Show that height of the cylinder of greatest volume which can be inscribed in a right circular cone of height h and semi vertical angle α is one-third that of the cone and the greatest volume of cylinder is 4π/27 (h3)tan α.
NCERT Solutions:
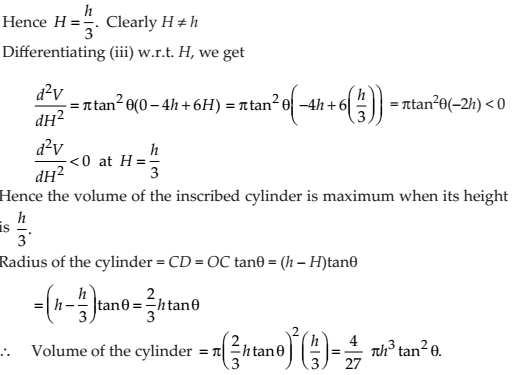
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 19.
A cylindrical tank of radius 10 m is being filled with wheat at the rate of 314 cubic metre per hour. Then the depth of the wheat is increasing at the rate of
(A) 1 m3/h (B) 0.1 m3/h (C) 1.1 m3/h (D) 0.5 m3/h
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 20.
The slope of the tangent to the curve x = t2 + 3t – 8, y = 2t2 – 2t – 5 at the point (2,– 1) is
(A) 22/7 (B)6/7 (C)7/6 (D) -6/7
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 21.
The line y = mx + 1 is a tangent to the curve y2 = 4x if the value of m is
(A) 1 (B) 2 (C) 3 (D) 1/2
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 22.
The normal at the point (1,1) on the curve 2y + x2 = 3 is
(A) x + y = 0 (B) x – y = 0 (C) x + y +1 = 0 (D) x – y = 0
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 23.
The normal to the curve x2 = 4y passing (1,2) is (A) x + y = 3 (B) x – y = 3 (C) x + y = 1 (D) x – y = 1
NCERT Solutions:
NCERT Solutions for Class 12 Maths Application of Derivatives Miscellaneous Exercise: Ques No 24.
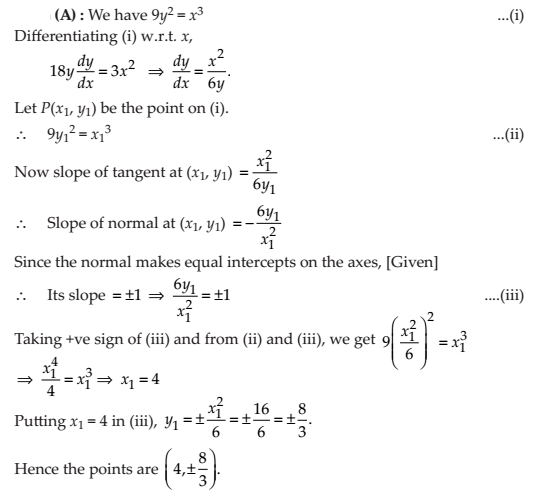
The points on the curve 9y2 = x3, where the normal to the curve makes equal intercepts with the axes are
NCERT Solutions: